Mathematics (Grades 5–12)
Subtest 1 Sample Items
Recommendation for individuals using a screenreader: please set your punctuation settings to "most."
Expand All | Collapse All
Question 1
1. The variables x and y represent real numbers and x + 2y − xi + 6i = 5 − 7i, what is
x − y?
1. The variables x and y represent real numbers and x plus 2 y minus x i plus 6 i equals 5
minus 7 i. What is x minus y?
- –9negative 9
- –4negative 4
- 2
- 17
Answer to question 1
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0001) First, solve for x
and y by rewriting the equation as (x + 2y) + (−x + 6)i = 5 − 7i. Separate the real terms and the imaginary terms to form a system of equations: x + 2y = 5 and (−x + 6)i = −7i. Obtain the value of x, 13, from the second equation and substitute this into the first equation to obtain y = −4. It follows that x − y = 17.
Correct Response: D. (Objective 0001) First, solve for x and y by rewriting the equation
as open parenthesis x plus two y close parenthesis plus open parenthesis negative x plus six close parenthesis
i equals five minus seven i. Separate the real terms and the imaginary terms to form a system of equations: x plus two y equals five and open parenthesis negative x plus six close parenthesis i equals negative seven i. Obtain the value of x, 13, from the second equation and substitute this into the first equation to obtain y equals negative 4. It follows that x minus y equals 17.
Question 2
2. If (2012)3subscript 3 =
(3a3)4subscript 4,
what is the value of a?
- 1
- 2
- 4
- 8
Answer to question 2
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0001) One way to approach
this problem is to convert both numbers to base ten: (2012)3 = 2 × 33 +
0 × 32 + 1 × 31 + 2 × 30 = (54 + 0 + 3 + 2)10
= (59)10 and (3a3)4 = 3 × 42 + a × 41
+ 3 × 40 = (48 + 4a + 3)10 = (51 + 4a)10. Thus,
(51 + 4a)10 = (59)10
 4a = 8
4a = 8
 a = 2.
Correct Response: B. (Objective 0001) One way to approach this problem is to convert
both numbers to base ten: open parenthesis 2012 close parenthesis subscript three equals 2 multiplied
by 3 cubed plus 0 multiplied by 3 squared plus 1 multiplied by 3 superscript one plus 2 multiplied by
3 sup zero equals open parenthesis 54 plus 0 plus 3 plus 2 close parenthesis sub ten equals open parenthesis
59 close parenthesis subscript ten and open parenthesis 3 a 3 close parenthesis subscript four
equals 3 multiplied by 4 squared plus a multiplied by 4 superscript one plus 3 multiplied by
4 superscript zero equals open parenthesis 48 plus 4 a plus 3 close parenthesis subscript ten
equals open parenthesis 51 plus 4 a close parenthesis. Thus, open parenthesis 51 plus 4 a
close parenthesis subscript ten equals open parenthesis 59 close parenthesis sub ten leads to 4 a
equals 8 rightwards double arrow a equals 2.
a = 2.
Correct Response: B. (Objective 0001) One way to approach this problem is to convert
both numbers to base ten: open parenthesis 2012 close parenthesis subscript three equals 2 multiplied
by 3 cubed plus 0 multiplied by 3 squared plus 1 multiplied by 3 superscript one plus 2 multiplied by
3 sup zero equals open parenthesis 54 plus 0 plus 3 plus 2 close parenthesis sub ten equals open parenthesis
59 close parenthesis subscript ten and open parenthesis 3 a 3 close parenthesis subscript four
equals 3 multiplied by 4 squared plus a multiplied by 4 superscript one plus 3 multiplied by
4 superscript zero equals open parenthesis 48 plus 4 a plus 3 close parenthesis subscript ten
equals open parenthesis 51 plus 4 a close parenthesis. Thus, open parenthesis 51 plus 4 a
close parenthesis subscript ten equals open parenthesis 59 close parenthesis sub ten leads to 4 a
equals 8 rightwards double arrow a equals 2.
Question 3
3. Performing which of the following operations will simplify the fraction
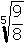 by rationalizing the denominator?
by rationalizing the denominator?
-

-

-

-

Answer to question 3
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0001) Rationalizing the denominator
requires eliminating the radical in the denominator. Express
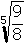 as
as
 . Then
. Then
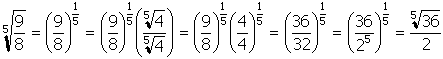 .
Correct Response: D. (Objective 0001) Rationalizing the denominator requires eliminating
the radical in the denominator. Express start fifth root nine eighths end fifth root as open parenthesis
nine eighths close parenthesis superscript one fifth . Then start fifth root nine eighths end fifth
root equals open parenthesis nine eighths close parenthesis superscript one fifth equals open parenthesis
nine eighths close parenthesis superscript one fifth baseline open parenthesis start fraction numerator
fifth root of four denominator fifth root of four end fraction close parenthesis equals open parenthesis
nine eights close parenthesis superscript one fifth baseline open parenthesis four over four close parenthesis
superscript one fifth equals open parenthesis thirty six over thirty two close parenthesis superscript
one fifth equals open parenthesis thirty six over two superscript five close parenthesis superscript
one fifth equals start fraction numerator fifth root of thirty six denominator two end fraction.
.
Correct Response: D. (Objective 0001) Rationalizing the denominator requires eliminating
the radical in the denominator. Express start fifth root nine eighths end fifth root as open parenthesis
nine eighths close parenthesis superscript one fifth . Then start fifth root nine eighths end fifth
root equals open parenthesis nine eighths close parenthesis superscript one fifth equals open parenthesis
nine eighths close parenthesis superscript one fifth baseline open parenthesis start fraction numerator
fifth root of four denominator fifth root of four end fraction close parenthesis equals open parenthesis
nine eights close parenthesis superscript one fifth baseline open parenthesis four over four close parenthesis
superscript one fifth equals open parenthesis thirty six over thirty two close parenthesis superscript
one fifth equals open parenthesis thirty six over two superscript five close parenthesis superscript
one fifth equals start fraction numerator fifth root of thirty six denominator two end fraction.
Question 4
4. If the expression
 represents vector v in the complex plane, what are the polar coordinates of v?
represents vector v in the complex plane, what are the polar coordinates of v?
-
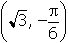
-
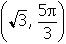
-
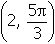
-
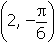
Answer to question 4
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0001) The rectangular coordinates
of
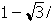 are
are
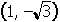 ,
placing it in Quadrant IV. Polar coordinates are of the form (r, θ
), where r represents the radial distance of
,
placing it in Quadrant IV. Polar coordinates are of the form (r, θ
), where r represents the radial distance of
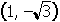 from the origin, calculated as
from the origin, calculated as
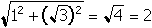 by application of the Pythagorean theorem, and θ represents the polar
angle of v in Quadrant IV, calculated by using reference angle
by application of the Pythagorean theorem, and θ represents the polar
angle of v in Quadrant IV, calculated by using reference angle
 ;
thus the polar coordinates of v are
;
thus the polar coordinates of v are
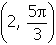 .
Correct Response: C. (Objective 0001) The rectangular coordinates of one minus
square root of three are open parenthesis one comma negative square root of three close parenthesis,
placing it in Quadrant I V. Polar coordinates are of the form open parenthesisr, theta close
parenthesis, where r represents the radial distance of open parenthesis one comma negative
square root of three close parenthesis from the origin, calculated as start square root one squared
plus open parenthesis square root of three close parenthesis squared end square root equals square root
of four equals two by application of the Pythagorean theorem, and theta represents the polar angle of
v in Quadrant I V, calculated by using reference angle arc tangent square root of three
equals pi over six; thus the polar coordinates of v are open parenthesis two comma
five pi over three close parenthesis.
.
Correct Response: C. (Objective 0001) The rectangular coordinates of one minus
square root of three are open parenthesis one comma negative square root of three close parenthesis,
placing it in Quadrant I V. Polar coordinates are of the form open parenthesisr, theta close
parenthesis, where r represents the radial distance of open parenthesis one comma negative
square root of three close parenthesis from the origin, calculated as start square root one squared
plus open parenthesis square root of three close parenthesis squared end square root equals square root
of four equals two by application of the Pythagorean theorem, and theta represents the polar angle of
v in Quadrant I V, calculated by using reference angle arc tangent square root of three
equals pi over six; thus the polar coordinates of v are open parenthesis two comma
five pi over three close parenthesis.
Question 5
5. Which of the following functions has zeros of 1 and i ?
- f(x) = x3 − x2
+ x − 1f of x = x cubed minus x squared plus x minus 1
- f(x) = x3 + x2 +
x − 1f of x = x cubed plus x squared plus x minus 1
- f(x) = x3 − x2
− x − 1f of x = x cubed minus x squared minus x minus
1
- f(x) = x3 + x2 −
x − 1f of x = x cubed plus x squared minus x minus 1
Answer to question 5
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0001) Complex solutions to
polynomial equations with real-number coefficients occur in conjugate pairs; that is, if a
+ bi is a zero, then a − bi is also a zero. The desired polynomial
can be constructed by finding the product of its three known factors. Thus, (x − 1)(x − i)(x + i) = (x − 1)(x2 − i2) = (x − 1)(x2 + 1) = x3
− x2 + x − 1.
Correct Response: A. (Objective 0001) Complex solutions to polynomial equations
with real-number coefficients occur in conjugate pairs; that is, if a + b i is a zero,
then a minus b i is also a zero. The desired polynomial can be constructed by finding
the product of its three known factors. Thus, open parenthesis x minus one close parenthesis open parenthesis
x minus i close parenthesis open parenthesis plus i close parenthesis equals open parenthesis x minus
one close parenthesis open parenthesis x squared minus i squared close parenthesis equals open parenthesis
x minus one close parenthesis open parenthesis x squared plus one close parenthesis equals x cubed minus
x squared plus x minus one.
Question 6
6. If the numerator n has no factors in common with each of its respective denominators, which
of the following fractions can be written as a terminating decimal?
- n/150n over 150
- n/160n over one 160
- n/220n over 220
- n/230n over 230
Answer to question 6
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0001) Terminating decimals
have fractional representations whose denominators have prime factorizations that can be written as
powers of only 2, 5, or both 2 and 5. The denominator 160 = 25 × 5, so n/160n over one 160
can be expressed as a terminating decimal.
Correct Response: B. (Objective 0001) Terminating decimals have fractional representations
whose denominators have prime factorizations that can be written as powers of only two, five, or both
two and five. The denominator one hundred sixty equals two to the fifth power times five, so n over
one hundred sixty can be expressed as a terminating decimal.
Question 7
7. Three strategies for adding a column of one hundred integers are shown below:
- Compute a sum for each set of five integers, then add all of the partial sums.
- Sort the integers by size, then compute a sum for each subset of identical integers and add all of
the partial sums.
- Add each consecutive number to a running sum until all of the numbers have been added.
All three strategies are valid because the:
- set of integers contains an additive inverse for each integer.
- addition of integers distributes over multiplication.
- set of integers is closed under the operation of addition.
- addition of integers has associative and commutative properties.
Answer to question 7
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0002) The associative property
of addition allows the grouping of the addends to be changed, and the commutative property of addition
allows the order of the addends to be changed without changing the result. Each of the described strategies
is based on one or both of these properties.
Correct Response: D. (Objective 0002) The associative property of addition allows
the grouping of the addends to be changed, and the commutative property of addition allows the order
of the addends to be changed without changing the result. Each of the described strategies is based
on one or both of these properties.
Question 8
8. Two numbers, x and y, have a greatest common factor of 22
• 33 • 52two squared times three cubed times
five squared and a least common multiple of 24 • 34
• 53two superscript four times three superscript four times
five superscript three. If y = 22 • 34
• 53y equals two squared times three exponent 4 times 5
superscript three, which of the following factorizations represents x?
- 22 • 31 • 51two
squared times three superscript one times five superscript one
- 22 • 33 • 53two
squared times three superscript three times five superscript three
- 24 • 34 • 53two
superscript four times three superscript four times five superscript three
- 24 • 33 • 52two
superscript four times three cubed times five squared
Answer to question 8
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0002) The greatest common
factor is the greatest whole number that is a factor of both x and y. Since 33
represents the largest exponential representation of 3 that is a factor of both x and y,
33 must be present in the prime factorization of x (y includes 34
as a factor). Similar reasoning shows that 52 must be a factor of x. The least common
multiple is the least whole number that is a multiple of both x and y. Since 24
represents the least exponential representation of 2 that is a multiple of both x and y,
and y includes only 22 as a factor, 24 must be present in the prime factorization
of x. Thus, 24 • 33 • 52 is correct.
Correct Response: D. (Objective 0002) The greatest common factor is the greatest
whole number that is a factor of both x and y. Since 3 cubed represents the largest
exponential representation of 3 that is a factor of both x and y, 3 cubed must be
present in the prime factorization of x (y includes 3 sup four as a factor). Similar
reasoning shows that 5 squared must be a factor of x. The least common multiple is the least
whole number that is a multiple of both x and y. Since 2 sup four represents the least
exponential representation of 2 that is a multiple of both x and y, and y
includes only 2 squared as a factor, 2 superscript four must be present in the prime factorization of
x. Thus, 2 superscript four times 3 cubed times 5 squared is correct.
Question 9
9. Given the relative positions of a, b, c, and d on the number
line below, which of the following fractions has the smallest value?
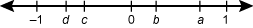
intervals are unevenly spaced. 0 is approximately in the center. Moving to the right of 0, b is approximately
one-quarter distance away from 0, a is approximately two quarters distance away from b, and 1 is approximately
one quarter distance away from a. Moving to the left of 0, c is approximatelt two quarters away from
zero, d is less than one quarter away from c, and -1 is greater than one quarter away from d.
- d/cd over c
- b/ab over a
- d/bd over b
- a/ca over c
Answer to question 9
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0002) Note that a,
b, c, and d are all fractions between 1 and −1. Of the response options
provided, only
d/bd over b
and
a/ca over c
are negative, so one of them must be the correct answer. Since the numerators d and a
appear to have equal absolute values, the fraction with the smaller absolute value in the denominator
will give the answer farthest to the left on the number line, i.e.,
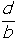 .
Correct Response: C. (Objective 0002) Note that a, b, c,
and d are all fractions between 1 and negative 1. Of the response options provided, only d
over b and a over c are negative, so one of them must be the correct answer. Since the numerators d
and a appear to have equal absolute values, the fraction with the smaller absolute value in
the denominator will give the answer farthest to the left on the number line, i.e., d over b.
.
Correct Response: C. (Objective 0002) Note that a, b, c,
and d are all fractions between 1 and negative 1. Of the response options provided, only d
over b and a over c are negative, so one of them must be the correct answer. Since the numerators d
and a appear to have equal absolute values, the fraction with the smaller absolute value in
the denominator will give the answer farthest to the left on the number line, i.e., d over b.
Question 10
10. In the problem solution below, in what order are the operational properties applied?
Step 1: 4 − [a + 6]
Step 2: 4 − 1[a + 6]
Step 3: 4 + (−1)[a + 6]
Step 4: 4 + [(−a) + (−6)]
Step 5: 4 + [(−6) + (−a)]
Step 6: [4 + (−6)] + (−a)
Step 7: −2 + (−a)
Step 8: −2 − a Step 1: 4 minus left
bracket a plus 6 right bracket
Step 2: 4 minus 1 left bracket a plus 6 right bracket
Step 3: 4 plus open parenthesis negative 1 close parenthesis left bracket a plus 6 right bracket
Step 4: 4 plus left bracket open parenthesis negative a close parenthesis plus open parenthesis
negative 6 close parenthesis right bracket
Step 5: 4 plus left bracket open parenthesis negative 6 close parenthesis plus open parenthesis negative
a close parenthesis right bracket
Step 6: left bracket 4 plus open parenthesis negative 6 close parenthesis right bracket plus open parenthesis
negative a close parenthesis
Step 7: negative 2 plus open parenthesis negative a close parenthesis
Step 8: negative 2 minus a
- associative, distributive, commutative
- distributive, commutative, associative
- associative, commutative, distributive
- distributive, associative, commutative
Answer to question 10
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0002) Rewriting the expression
(−1)[a + 6] as [(−a) + (−6)] between steps 3 and 4 is justified
by the fact that multiplication distributes over addition. Rearranging the order of the addition [(−a)
+ (−6)] to [(−6) + (−a)] between steps 4 and 5 is justified by the commutative
property. Regrouping 4 + [(−6) + (−a)] as [4 + (−6)] + (−a)
between steps 5 and 6 is justified by the associative property.
Correct Response: B. (Objective 0002) Rewriting the expression open parenthesis
negative one close parenthesis left bracket a plus six right bracket as left bracket open parenthesis
negative a close parenthesis plus open parenthesis negative six close parenthesis right bracket between
steps three and four is justified by the fact that multiplication distributes over addition. Rearranging
the order of the addition left bracket open parenthesis negative a close parenthesis plus open parenthesis
negative six close parenthesis right bracket to left bracket open parenthesis negative six close parenthesis
plus open parenthesis negative a close parenthesis right bracket between steps four and five is justified
by the commutative property. Regrouping four plus left bracket open parenthesis negative six close parenthesis
plus open parenthesis negative a close parenthesis right bracket as left bracket four plus
open parenthesis negative six close parenthesis right bracket plus open parenthesis negative a
close parenthesis between steps five and six is justified by the associative property.
Question 11
11. If the number N is a perfect square, which of the following must be true?
-
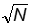 is a prime number.
is a prime number.
- If m is a factor of N, then m is a factor of
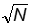 .
.
- When 1 and N are included, N has an odd number of factors.
- N equals the sum of all its factors less than N.
Answer to question 11
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0002) When listing factors,
notice that they can be listed in pairs. For example, the factors of 12 are 1 and 12, 2 and 6, and 3
and 4. Factors of a perfect square will pair the square root with itself: for 16, the factor pairs are
1 and 16, 2 and 8, and 4 and 4. But the pair 4, 4 does not represent 2 factors, so factors of 16 are
1, 2, 4, 8, and 16. This pattern is true for every perfect square, so perfect squares have an odd number
of factors.
Correct Response: C. (Objective 0002) When listing factors, notice that they can
be listed in pairs. For example, the factors of 12 are 1 and 12, 2 and 6, and 3 and 4. Factors of a
perfect square will pair the square root with itself: for 16, the factor pairs are 1 and 16, 2 and 8,
and 4 and 4. But the pair 4, 4 does not represent 2 factors, so factors of 16 are 1, 2, 4, 8, and 16.
This pattern is true for every perfect square, so perfect squares have an odd number of factors.
Question 12
12. If the heart rate of an average person living in North America is 70 beats per minute, which of the following estimates best approximates the total number of heartbeats in the full life span of that average person?
- 4.7 × 1074.7 times 10 to the power of 7
- 1.2 × 1081.2 times 10 to the power of 8
- 2.8 × 1092.8 times 10 to the power of 9
- 1.7 × 10111.7 times 10 to the power of 11
Answer to question 12
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0003) 70 beats/1 min. × 60 min./1 hr. × 24 hrs./1 day × 365 days/1 yr. × 75 yrs.= 2,759,400,000
≈ 2.8 × 109. Any reasonable estimate in the number of heartbeats per minute or
average life span per person will still round to the correct response.
Correct Response: C. (Objective 0003) 70 beats over 1 minute times 60
minutes over 1 hour times 24 hours over 1 day times 365 days over
1 year times 75 years equals 2,759,400,000 almost equal to 2.8 times 10 to the power of 9. Any reasonable estimate of the average life span per person will still round to the correct response.
Question 13
13. A nursery owner has a 40-pound bag of a native grass seed mixture. When 8 pounds of bluestem seeds
are added to this mixture, it becomes about 27% bluestem. Approximately what percent of the original
40-pound bag consisted of bluestem seed?
- 11.1%
- 12.4%
- 14.3%
- 16.7%
Answer to question 13
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0003) The amount of bluestem
seed, b, in the 40-pound bag, plus the 8 pounds of bluestem seed added later, together represent
27% of the 48 pounds in the final mixture. This can be written algebraically as b + 8/48 = 0.27
. Solving for b yields b =
4.96 pounds, which is exactly 12.4% of 40.
Correct Response: B. (Objective 0003) The amount of bluestem seed, b,
in the forty pound bag, plus the eight pounds of bluestem seed added later, together represent twenty
seven percent of the forty eight pounds in the final mixture. This can be written algebraically as start
fraction numerator b plus eight denominator forty eight end fraction equals zero point two seven. Solving
for b yields b equals four point nine six pounds, which is exactly twelve point four
percent of forty.
Question 14
14. A group consisting of 3 adults, 5 teenagers, and 4 young children stops at a restaurant on the way
home from a soccer tournament. Rather than figuring out each person's share of the final bill exactly,
they decide that a teenager's share would be
3/53 fifths
of an adult's share, and each child's share would be
1/31 third
of an adult's share. If the total cost of the meal was $66, what was the share of the family that consisted
of an adult and two young children?
- $13.50
- $15.00
- $16.50
- $18.00
Answer to question 14
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0003) The situation can be
represented algebraically by letting A represent an adult share. Thus,
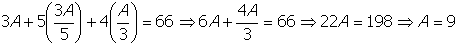 . The share of the bill for an adult and two
children was
. The share of the bill for an adult and two
children was
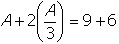 or $15.00.
Correct Response: B. (Objective 0003) The situation can be represented algebraically
by letting A represent an adult share. Thus, three a plus five open parenthesis three a over
five close parenthesis plus four open parenthesis a over three close parenthesis equals sixty six leads
to six a plus four a over three equals sixty six leads to twenty two a equals one hundred and ninety
eight leads to a equals nine. The share of the bill for an adult and two children was a plus two open
parenthesis a over three close parenthesis equals nine plus six or fifteen dollars.
or $15.00.
Correct Response: B. (Objective 0003) The situation can be represented algebraically
by letting A represent an adult share. Thus, three a plus five open parenthesis three a over
five close parenthesis plus four open parenthesis a over three close parenthesis equals sixty six leads
to six a plus four a over three equals sixty six leads to twenty two a equals one hundred and ninety
eight leads to a equals nine. The share of the bill for an adult and two children was a plus two open
parenthesis a over three close parenthesis equals nine plus six or fifteen dollars.
Question 15
15. An area diagram is an appropriate strategy for solving which of the following problems?
- What is the digit in the units place in the value of 3100?
- A child's piggy bank contains $6.70 in dimes and quarters. There are 40 coins in the bank. How many
are dimes?
- When 12 gallons of liquid are added to a tank that is
1/41 fourths
full, the tank will be
5/85 eighths
full. What is the capacity of the tank?
- A circular arrangement of flowers, evenly spaced and in numerical order, is on the front of a large
quilt. Flower 6 is directly opposite Flower 14. How many flowers are in the circle?
Answer to question 15
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0003) An area diagram can
be used to find the capacity of the tank. Let the total area of a rectangle represent the capacity of
the tank. Shade
2/8
to represent the initial amount in the tank and shade an additional
3/8
to obtain the
5/8
that represents the amount after adding 12 gallons. These 3 blocks of additional shading represent the
12 gallons, so each block depicts 4 gallons. Since the total area of the rectangle contains 8 blocks,
the capacity of the tank is 32 gallons.
Correct Response: C. (Objective 0003) An area diagram can be used to find the capacity
of the tank. Let the total area of a rectangle represent the capacity of the tank. Shade two eighths
to represent the initial amount in the tank and shade an additional three eighths to obtain the five
eighths that represents the amount after adding twelve gallons. These three blocks of additional shading
represent the twelve gallons, so each block depicts four gallons. Since the total area of the rectangle
contains eight blocks, the capacity of the tank is thirty two gallons.
Question 16
16. The velocity of a thin stream of water flowing from a small hole in the side of a tank is directly
proportional to the square root of the depth of the hole below the surface of the water. If the velocity
of the stream is 24 feet per second when the depth of the hole is 9 feet, what is the velocity of the
stream when the depth is 16 feet?
- 32.0 feet per second
- 42.7 feet per second
- 64.0 feet per second
- 75.9 feet per second
Answer to question 16
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0003) The velocity is directly
proportional to the square root of the depth. Let V represent velocity and d represent
depth. Then
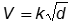 ,
where k is a constant of proportionality. Substituting,
,
where k is a constant of proportionality. Substituting,
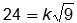 so k = 8. When the depth of the hole is 16 feet,
so k = 8. When the depth of the hole is 16 feet,
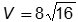 ,
which is 32 feet per second.
Correct Response: A. (Objective 0003) The velocity is directly proportional to
the square root of the depth. Let V represent velocity and d represent depth. Then
V equals k square root of d, where k is a constant of proportionality. Substituting, twenty
four equals k square root of nine so k equals eight. When the depth of the hole is sixteen
feet, V equals eight square root of sixteen, which is thirty two feet per second.
,
which is 32 feet per second.
Correct Response: A. (Objective 0003) The velocity is directly proportional to
the square root of the depth. Let V represent velocity and d represent depth. Then
V equals k square root of d, where k is a constant of proportionality. Substituting, twenty
four equals k square root of nine so k equals eight. When the depth of the hole is sixteen
feet, V equals eight square root of sixteen, which is thirty two feet per second.
Question 17
17. Which of the following equations corresponds to the data in the table below?
|
x
|
y
|
|
−negative3
|
12
|
|
−negative2
|
6
|
|
−negative1
|
4
|
|
0
|
6
|
|
2
|
22
|
- y – 4 = 2(x + 1)²y
minus four equals two open parenthesis x plus one close parenthesis the quantity squared
- y – 4 = 2(x – 1)²y
minus four equals two open parenthesis x minus one close parenthesis the quantity squared
- y + 4 = 2(x – 1)²y
plus four equals two open parenthesis x minus one close parenthesis the quantity squared
- y + 4 = 2(x + 1)²y plus
four equals two open parenthesis x plus one close parenthesis the quantity squared
Answer to question 17
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0004) The equation y
− k = a (x − h )2 represents the graph of
a parabola whose vertex is the point (h, k). The pattern of the data indicates that the vertex
is the lowest point (–1, 4), so h = –1 and k = 4. Since a = 2 in each
response, the parabola has the equation y − 4 = 2(x − –1)2,
which is equivalent to y − 4 = 2(x + 1)2.
Correct Response: A. (Objective 0004) The equation y minus k equals a open parenthesis
x minus h close parenthesis squared represents the graph of a parabola whose vertex is the point h comma
k. The pattern of the data indicates that the vertex is the lowest point negative one comma four, so
h equals negative one and k equals four. Since a equals two in each response, the parabola has the equation
y minus four equals two open parenthesis x minus negative one close parenthesis squared which is equivalent
to y minus four equals two open parenthesis x plus one close parenthesis squared.
Question 18
18. Which of the following functions corresponds to the points in the table below?
|
x
|
y
|
|
−negative3
|
−negative2
|
|
−negative2
|
−negative0.5
|
|
−negative1
|
0
|
|
0
|
0.25
|
|
1
|
0.4
|
|
2
|
0.5
|
-
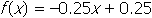
-
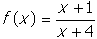
-
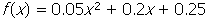
-
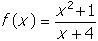
Answer to question 18
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0004) One way to determine
which function corresponds to the points in the table is to substitute values for x into each
of the answer choices to determine if the corresponding value of y is obtained. Notice that
substituting x = 0 into each of the functions results in 0.25 for all of the answer choices.
Substituting x = 1 eliminates f(x) = −0.25x + 0.25 and f(x)
= 0.05x2 + 0.2x + 0.25. Substituting x = 2 in the response choices
eliminates
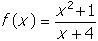 . Substituting −1, −2, and −3
in
. Substituting −1, −2, and −3
in
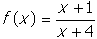 confirms that this function corresponds to the points in the table.
Correct Response: B. (Objective 0004) One way to determine which function corresponds
to the points in the table is to substitute values for x into each of the answer choices to
determine if the corresponding value of y is obtained. Notice that substituting x
equals zero into each of the functions results in zero point two five for all of the answer choices.
Substituting x equals one eliminates f of x equals negative zero point two five x plus zero
point two five and f of x equals zero point zero five x squared plus zero point two x plus zero point
two five. Substituting x equals two in the response choices eliminates f of x equals start fraction
numerator x squared plus one denominator x plus four end fraction. Substituting negative one, negative
two, and negative three in f of x equals start fraction numerator x plus one denominator x plus four
end fraction confirms that this function corresponds to the points in the table.
confirms that this function corresponds to the points in the table.
Correct Response: B. (Objective 0004) One way to determine which function corresponds
to the points in the table is to substitute values for x into each of the answer choices to
determine if the corresponding value of y is obtained. Notice that substituting x
equals zero into each of the functions results in zero point two five for all of the answer choices.
Substituting x equals one eliminates f of x equals negative zero point two five x plus zero
point two five and f of x equals zero point zero five x squared plus zero point two x plus zero point
two five. Substituting x equals two in the response choices eliminates f of x equals start fraction
numerator x squared plus one denominator x plus four end fraction. Substituting negative one, negative
two, and negative three in f of x equals start fraction numerator x plus one denominator x plus four
end fraction confirms that this function corresponds to the points in the table.
Question 19
19. A sequence of figures is shown below.
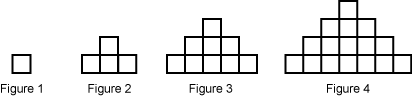
Figure one is a single square. Figure two has two rows of stacked squares, top row has one square and
bottom row has three squares. Figure three has three rows of stacked squares, top row has one square
second row has three squares and the bottom row has five squares. Figure four has four rows of stacked
squares, top row has one square, second row has three squares, third row has five squares and the fourth
row has seven squares.
In the following expressions, Tnsubscript
n represents the number of squares in the nth figure and nth term of
the sequence. If the pattern continues, which of the following expressions represents Tnsubscript n + 1?
- 2Tnsubscript n +
2
- Tnsubscript n +
(2n − 1)
- Tnsubscript n +
(2n + 1)
- 2Tnsubscript n +
2(2n − 1)
Answer to question 19
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0004) A recursive sequence
is a sequence in which later terms of the sequence can be deduced from previous terms. From analyzing
the pattern of squares in each figure of the sequence, it can be seen that Tn =
n2. Therefore, Tn + 1 = (n + 1)2
= n2 + 2n + 1. And since n2 = Tn,
Tn + 1 = Tn + (2n + 1).
Correct Response: C. (Objective 0004) A recursive sequence is a sequence in which
later terms of the sequence can be deduced from previous terms. From analyzing the pattern of squares
in each figure of the sequence, it can be seen that t subscript n equals n squared. Therefore, t subscript
n plus one baseline equals open parenthesis n plus one close parenthesis squared equals n squared plus
two n plus one. And since n squared equals t subscript n baseline comma t sub n plus one baseline equals
t subscript n baseline plus open parenthesis two n plus one close parenthesis.
Question 20
20. Given that f(x) = x − 1, g(x) = x2
+ 2x + 1, and h(x) = g(f(x)), which of the following
statements about h(x) is true?
- h(x) = h (−negativex)
- h(a + b) = h(a) + h(b)
- h(x) is one-to-one.
- h(x) = 0 has two distinct real solutions.
Answer to question 20
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0004) The composite
function h(x) = g(f(x)) = g(x −
1) = (x − 1)2 + 2(x − 1) + 1 = x2 −
2x + 1 + 2x − 2 + 1 = x2. Therefore, h(x)
= x2 and h(x) = h(−x).
Correct Response: A. (Objective 0004) The composite function h open parenthesis
x close parenthesis equals g open parenthesis f open parenthesis x close parenthesis close parenthesis
equals g open parenthesis x minus one close parenthesis equals open parenthesis x minus one close parenthesis
squared plus two open parenthesis x minus one close parenthesis plus one equals x squared minus two
x plus one plus two x minus two plus one equals x squared. Therefore, h open parenthesis x close parenthesis
equals x squared and h open parenthesis x close parenthesis equals h open parenthesis negative x close
parenthesis.
Question 21
21. Given that
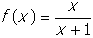 for x ≠ −not equal to negative1,
which of the following functions represents f−1(x) f superscript negative 1 of x, the inverse of
f(x) f of x ?
for x ≠ −not equal to negative1,
which of the following functions represents f−1(x) f superscript negative 1 of x, the inverse of
f(x) f of x ?
-
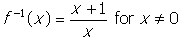
-
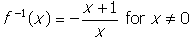
-
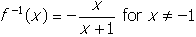
-
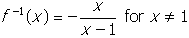
Answer to question 21
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0004) Since f(x)
is one-to-one on its domain, it has a unique inverse. If a function is represented by a set of ordered
pairs (x, y), then its inverse function is represented by the ordered pairs (y,
x). It follows that one way to find the inverse of the function
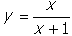 is to solve the equation
is to solve the equation
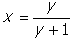 for y: x(y + 1) = y
for y: x(y + 1) = y
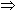 xy + x = y
xy + x = y
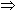 xy = y − x
xy = y − x
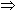 xy − y = −x
xy − y = −x
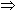 y(x − 1) = −x. Thus,
y(x − 1) = −x. Thus,
 and
and
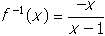 . This function is undefined at x =
1.
Correct Response: D. (Objective 0004) Since f of x is one-to-one on its
domain, it has a unique inverse. If a function is represented by a set of ordered pairs open parenthesis
x comma y close parenthesis, then its inverse function is represented by the ordered
pairs open parenthesis y comma x close parenthesis. It follows that one way to find
the inverse of the function y equals start fraction numerator x denominator x plus one end fraction
is to solve the equation x equals start fraction numerator y denominator y plus one end fraction for
y colon x open parenthesis y plus one close parenthesis equals y
leads to x y plus x equals y leads to x y equals y minus
x leads to x y minus y equals negative x leads to y parenthesis
x minus one close parenthesis equals negative x. Thus, y equals start fraction numerator
negative x denominator x minus one and inverse of f of x equals start fraction numerator negative x
denominator x minus one end fraction. This function is undefined at x equals one.
. This function is undefined at x =
1.
Correct Response: D. (Objective 0004) Since f of x is one-to-one on its
domain, it has a unique inverse. If a function is represented by a set of ordered pairs open parenthesis
x comma y close parenthesis, then its inverse function is represented by the ordered
pairs open parenthesis y comma x close parenthesis. It follows that one way to find
the inverse of the function y equals start fraction numerator x denominator x plus one end fraction
is to solve the equation x equals start fraction numerator y denominator y plus one end fraction for
y colon x open parenthesis y plus one close parenthesis equals y
leads to x y plus x equals y leads to x y equals y minus
x leads to x y minus y equals negative x leads to y parenthesis
x minus one close parenthesis equals negative x. Thus, y equals start fraction numerator
negative x denominator x minus one and inverse of f of x equals start fraction numerator negative x
denominator x minus one end fraction. This function is undefined at x equals one.
Question 22
22. The second term of a geometric sequence is
5/125 twelfths
and the sixth term is
20/320 thirds. What is the value
of the eighth term?
- 80/380 thirds
- 77/1277 twelfths
- 25/925 ninths
- 53/2453 twenty fourths
Answer to question 22
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0004) A geometric sequence
is formed when each term is multiplied by a particular constant to yield the next term. Let r
be the constant multiplier. Then
5/12•r•r•r•r = 20/3,
r4 = 16, and r = 2. Then 20/3•2•2 = 80/3.
Correct Response: A. (Objective 0004) A geometric sequence is formed when each
term is multiplied by a particular constant to yield the next term. Let r be the constant multiplier.
Then five twelfths times r times r times r times r equals twenty thirds, r to the fourth power equals
sixteen, and r equals two. Then twenty thirds times two times two equals eighty thirds.
Question 23
23. Which of the following expressions is equivalent to
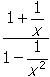 ?
?
-
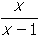
-
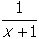
-

-
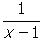
Answer to question 23
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0005)
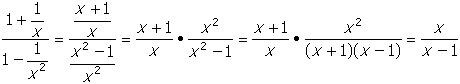 Correct Response: A. (Objective 0005) start fraction numerator one plus one over
x denominator one minus one over x squared end fraction equals start fraction numerator start fraction
numerator x plus one denominator x end fraction denominator start fraction numerator x squared minus
one denominator x squared end fraction equals start fraction numerator x plus one denominator x end
fraction times start fraction numerator x squared denominator x squared minus one end fraction equals
start fraction numerator x plus one denominator x end fraction time start fraction numerator x squared
denominator open parenthesis x plus one close parenthesis open parenthesis x minus one close parenthesis
end fraction equals start fraction numerator x denominator x minus one end fraction.
Correct Response: A. (Objective 0005) start fraction numerator one plus one over
x denominator one minus one over x squared end fraction equals start fraction numerator start fraction
numerator x plus one denominator x end fraction denominator start fraction numerator x squared minus
one denominator x squared end fraction equals start fraction numerator x plus one denominator x end
fraction times start fraction numerator x squared denominator x squared minus one end fraction equals
start fraction numerator x plus one denominator x end fraction time start fraction numerator x squared
denominator open parenthesis x plus one close parenthesis open parenthesis x minus one close parenthesis
end fraction equals start fraction numerator x denominator x minus one end fraction.
Question 24
24. Finding x in the equation ℓnatural
log n(x −minus
5) + ℓnatural log n(x
+ 2) = 3 ℓnatural log n 2 by using
algebraic techniques involves solving which of the following quadratic equations?
- x2x squared −minus 3x
−minus 18 = 0
- x2x squared −minus 3x
−minus 24 = 0
- x2x squared −minus 2x
−minus 18 = 0
- x2x squared −minus 2x
−minus 24 = 0
Answer to question 24
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0005) ℓn(x
− 5) + ℓn(x + 2) = 3 ℓn 2
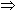 ℓn(x − 5)(x + 2) = ℓn 23
ℓn(x − 5)(x + 2) = ℓn 23
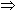 ℓn(x − 5)(x + 2) = ℓn 8
ℓn(x − 5)(x + 2) = ℓn 8
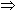 (x − 5)(x + 2) = 8
(x − 5)(x + 2) = 8
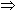 x2 − 3x − 10 = 8
x2 − 3x − 10 = 8
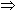 x2 − 3x − 18 = 0.
Correct Response: A. (Objective 0005) natural log open parenthesis x minus five
close parenthesis plus natural log open parenthesis x plus two close parenthesis equals natural log
two leads to natural log open parenthesis x minus five close parenthesis open parenthesis x plus two
close parenthesis equals natural log two cubed leads to natural log open parenthesis x minus five close
parenthesis open parenthesis x plus two close parenthesis equals natural log eight leads to open parenthesis
x minus five close parenthesis open parenthesis x plus two close parenthesis equals eight leads to x
squared minus three x minus ten equals eight leads to x squared minus three x minus eighteen equals
zero.
x2 − 3x − 18 = 0.
Correct Response: A. (Objective 0005) natural log open parenthesis x minus five
close parenthesis plus natural log open parenthesis x plus two close parenthesis equals natural log
two leads to natural log open parenthesis x minus five close parenthesis open parenthesis x plus two
close parenthesis equals natural log two cubed leads to natural log open parenthesis x minus five close
parenthesis open parenthesis x plus two close parenthesis equals natural log eight leads to open parenthesis
x minus five close parenthesis open parenthesis x plus two close parenthesis equals eight leads to x
squared minus three x minus ten equals eight leads to x squared minus three x minus eighteen equals
zero.
Question 25
25. A matrix multiplication problem is shown below.
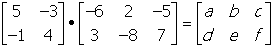
Start two by two matrix first row first column five second column negative three second row first column
negative one second column four end matrix times start two by three matrix first row first column negative
six second column two third column negative five second row first column three second column negative
eight third column seven end matrix equals start two by three matrix first row first column a second
column b third column c second row first column d second column e third column f end matrix.
Which of the following pairs of numbers in the product matrix has a sum of zero?
- a and c
- c and d
- b and e
- a and f
Answer to question 25
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. Objective 0005) The matrix on the left
is 2 × 2 and the matrix on the right is 2 × 3, so their product is a 2 ×
3 matrix. In a matrix, ti,j indicates the entry in row i and column j.
To find the entry for row i and column j in the product matrix, select row i
of the matrix on the left and column j of the matrix on the right, multiply the corresponding
row and column entries, and sum their products. For example, the entry t2,3 is calculated
as (−1)(−5) + (4)(7) = 33. Thus, the product matrix for the problem shown is
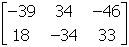 and it can be seen that entries b and e sum to 0.
Correct Response: C. Objective 0005) The matrix on the left is two by two and the
matrix on the right is two by three, so their product is a two by three matrix. In a matrix,
t subscript i comma j indicates the entry in row i and column j. To find
the entry for row i and column j in the product matrix, select row i of the
matrix on the left and column j of the matrix on the right, multiply the corresponding row
and column entries, and sum their products. For example, the entry t subscript two comma three
is calculated as open parenthesis negative one close parenthesis open parenthesis negative five plus
open parenthesis four close parenthesis open parenthesis seven close parenthesis equals thirty three.
Thus, the product matrix for the problem shown is Start two by three matrix first row first column negative
thirty nine second column thirty four third column negative forty six second row first column eighteen
second column negative thirty four third column thirty three end matrix and it can be seen that entries
b and e sum to zero.
and it can be seen that entries b and e sum to 0.
Correct Response: C. Objective 0005) The matrix on the left is two by two and the
matrix on the right is two by three, so their product is a two by three matrix. In a matrix,
t subscript i comma j indicates the entry in row i and column j. To find
the entry for row i and column j in the product matrix, select row i of the
matrix on the left and column j of the matrix on the right, multiply the corresponding row
and column entries, and sum their products. For example, the entry t subscript two comma three
is calculated as open parenthesis negative one close parenthesis open parenthesis negative five plus
open parenthesis four close parenthesis open parenthesis seven close parenthesis equals thirty three.
Thus, the product matrix for the problem shown is Start two by three matrix first row first column negative
thirty nine second column thirty four third column negative forty six second row first column eighteen
second column negative thirty four third column thirty three end matrix and it can be seen that entries
b and e sum to zero.
Question 26
26. A problem is solved using the steps shown below.
Line 1: x2 + 5x = −negative6
Line 2: x2 + 5x + 6 = 0
Line 3: (x + 3)(x + 2) = 0
Line 4: x + 3 = 0 or x + 2 = 0
Line 5: x = −negative3
or x = −negative2
Between which two lines is the distributive property applied?
- Line 1 and Line 2
- Line 2 and Line 3
- Line 3 and Line 4
- Line 4 and Line 5
Answer to question 26
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0005) Factoring an expression
is justified by the distributive property of multiplication over addition; working backward, (x
+ 3)(x + 2) = x • x + x • 2 + 3 • x + 3
• 2 = x2 + 5x + 6.
Correct Response: B. (Objective 0005) Factoring an expression is justified by the
distributive property of multiplication over addition; working backward, open parenthesis x plus three
close parenthesis open parenthesis x plus two close parenthesis equals x times x plus x times two plus
three times x plus three times two equals x squared plus five x plus six.
Question 27
27. What is R if 6x3 − 5x2+
15x − 5 = (3x − 1)(2x2 − x + 3) + R?
What is R if six x cubed minus five x squared plus fifteen x minus five
equals open parenthesis three x minus one closed parenthesis times open parenthesis two x squared minus
x plus three end parenthesis plus R?
- 2x − 8
- 5x − 2
- 7x + 2
- 9x + 8
Answer to question 27
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0005) This question can be
answered using either multiplication or long division. Using multiplication, (3x − 1)(2x2
− x + 3) + R = 6x3 − 3x2 + 9x
− 2x2 + x − 3 + R = 6x3 −
5x2 + 10x − 3 + R. Setting this result equal to 6x3
− 5x2 + 15x − 5, it can be shown that 10x −
3 + R = 15x − 5. Solving for R yields R = 5x − 2.
Correct Response: B. (Objective 0005) This question can be answered using either
multiplication or long division. Using multiplication, open parenthesis three x minus one end parenthesis
times open parenthesis two x squared minus x plus three end parenthesis plus R equals six x cubed minus
three x squared plus nine x minus two x squared plus x minus three plus R equals six x cubed minus five
x squared plus ten x minus three plus R. Setting this result equal to six x cubed minus five x squared
plus fifteen x minus five, it can be shown that ten x minus three plus R equals fifteen x minus five.
Solving for R yields R equals five x minus two.
Question 28
28. The demand for a certain commodity is given by x = –negative12(ℓn p − ℓn 120
natural log n of p minus natural log n of one hundred twenty)
where p represents the price per unit and x represents the number of units sold. Which
of the following equations expresses the price per unit as a function of the number of units sold?
-
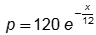
-
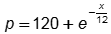
-
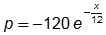
-
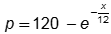
Answer to question 28
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0005) Solving for p:
x = –12(ℓn p − ℓn 120)
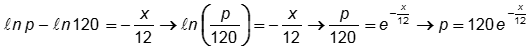 .
Correct Response: A. (Objective 0005) Solving for p: x equals negative twelve open
parenthesis l n of p minus l n of one hundred twenty end parenthesis, yields l n of p minus l n of one
hundred twenty equals negative one twelfth x, yields l n of p over one hundred twenty equals negative
one twelfth x, yields p over one hundred twenty equals e to the negative one twelfth x power, yields
p equals one hundred twenty e to the negative one twelfth x power.
.
Correct Response: A. (Objective 0005) Solving for p: x equals negative twelve open
parenthesis l n of p minus l n of one hundred twenty end parenthesis, yields l n of p minus l n of one
hundred twenty equals negative one twelfth x, yields l n of p over one hundred twenty equals negative
one twelfth x, yields p over one hundred twenty equals e to the negative one twelfth x power, yields
p equals one hundred twenty e to the negative one twelfth x power.
Question 29
29. The entrance to a tunnel has the shape of an arch that is modeled by a quadratic equation with roots
of –2negative two and 5 and a leading
coefficient of –1negative one.
Approximately how high in feet is the tunnel entrance at its highest point?
- 8 ft.8 feet
- 12 ft.12 feet
- 14 ft.14 feet
- 17 ft.17 feet
Answer to question 29
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0006) The form y
= a (x − r1)(x − r2)
represents a quadratic equation whose roots are r1 and r2 and
whose leading coefficient is a. Substituting the information given results in the model y =
–1(x − –2)(x − 5) or y = –1(x + 2)(x
− 5). The highest point will occur when the x value is halfway between –2 and 5, or x = 1.5. Substituting 1.5 for x in the equation results in y = –1(3.5)(–3.5)
= 12.25, or approximately 12 feet.
Correct Response: B. (Objective 0006) The form y equals a open parenthesis x minus
r subscript one close parenthesis open parenthesis x minus r subscript two close parentheses represents
a quadratic equation whose roots are r subscript one and r subscript two and whose leading coefficient
is a. Substituting the information given results in the model y equals negative one open parenthesis
x minus negative two close parenthesis open parenthesis x minus five close parenthesis or y equals negative
one open parenthesis x plus two close parenthesis open parenthesis x minus five close parenthesis. The highest point will occur when the x value is halfway between negative 2 and 5, or x equals 1 point 5. Substituting one point five for x in the equation results in y equals negative one open parenthesis
three point five close parenthesis open parenthesis negative three point five close parenthesis which
equals twelve point two five, or approximately twelve feet.
Question 30
30. When graphed, the vertex of which of the following functions lies on the x-axis?
- y = 2x2 + 3x + 1y
= 2x squared plus 3x plus 1
- y = −2x2 + 2x − 1y = negative 2x squared plus 2x minus 1
- y = −2x2 + 4x − 2y = negative 2x squared plus 4x minus 2
- y = 2x2 + 4x + 1y
= 2x squared plus 4x plus 1
Answer to question 30
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0006) The graph of a quadratic
function of the form y = ax2 + bx + c is a parabola with
the x-coordinate of its vertex at
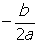 .
The equation y = −2x2
+ 4x − 2 has a vertex with an x-coordinate of
.
The equation y = −2x2
+ 4x − 2 has a vertex with an x-coordinate of
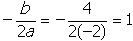 . Evaluating y at x = 1 yields
−2(1)2 + 4(1) − 2 = 0. Thus the parabola that represents y = −2x2
+ 4x − 2 has its vertex at the point (1, 0) on the x-axis.
Correct Response: C. (Objective 0006) The graph of a quadratic function of the
form y equals a x squared plus b x plus c is a parabola with the x-coordinate of its vertex
at negative start fraction numerator b denominator two a end fraction. The equation y equals negative two x squared plus four x minus two has a vertex with an x-coordinate
of negative start fraction numerator b denominator two a end fraction equals negative start fraction
numerator four denominator two open parenthesis negative two close parenthesis end fraction equals one.
Evaluating y at x equals one yields negative two open parenthesis one close parenthesis squared
plus four open parenthesis one close parenthesis minus two equals zero. Thus the parabola that represents
y equals negative two x squared plus four x minus two has its vertex at the point open parenthesis one
comma zero close parenthesis on the x-axis.
. Evaluating y at x = 1 yields
−2(1)2 + 4(1) − 2 = 0. Thus the parabola that represents y = −2x2
+ 4x − 2 has its vertex at the point (1, 0) on the x-axis.
Correct Response: C. (Objective 0006) The graph of a quadratic function of the
form y equals a x squared plus b x plus c is a parabola with the x-coordinate of its vertex
at negative start fraction numerator b denominator two a end fraction. The equation y equals negative two x squared plus four x minus two has a vertex with an x-coordinate
of negative start fraction numerator b denominator two a end fraction equals negative start fraction
numerator four denominator two open parenthesis negative two close parenthesis end fraction equals one.
Evaluating y at x equals one yields negative two open parenthesis one close parenthesis squared
plus four open parenthesis one close parenthesis minus two equals zero. Thus the parabola that represents
y equals negative two x squared plus four x minus two has its vertex at the point open parenthesis one
comma zero close parenthesis on the x-axis.
Question 31
31. Given the graph of y = f(x) below, the graph of
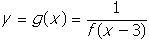 has a vertical asymptote at which of the following points?
has a vertical asymptote at which of the following points?
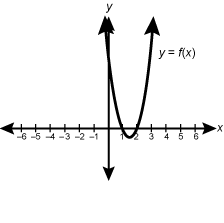
the vertex is in the fourth quadrant just below the x axis at some negative y value and one point five
for the x value. From the vertex it increases quickly to the left into the second quadrant passing the
y axis at some y value. From the vertex it increases quickly to the right in the first quadrant and
continues to increase.
- x = –negative 5
- x = –negative 2
- x = 3
- x = 4
Answer to question 31
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0006) The graph of function
g(x) is the reciprocal of function f(x), moved 3 units to the right
along the x-axis. Any point at which f(x) = 0 will represent a point at which
g(x) is undefined and has an asymptote. From the graph given, the zeros of f(x)
occur at x = 1 and x = 2. Moving them 3 units to the right will result in asymptotes
for g(x) at the points x = 4 and x = 5.
Correct Response: D. (Objective 0006) The graph of function g of x
is the reciprocal of function f of x, moved three units to the right along the x-axis. Any
point at which f of x equals zero will represent a point at which g of x is undefined and has
an asymptote. From the graph given, the zeros of f of x occur at x equals one and x
equals two. Moving them three units to the right will result in asymptotes for g of x at the points
x equals four and x equals five.
Question 32
32. The number of mosquitoes on a farm is estimated to be 500,000. Some bats are introduced to feed
on them and in 5 days the number of mosquitoes decreases to 300,000. If the number of mosquitoes is
decreasing exponentially, approximately how many mosquitoes will there be in 7 days?
- 245,000
- 220,000
- 180,000
- 108,000
Answer to question 32
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0006) The situation can be
modeled using an exponential function of the form N = N0 × rt,
where N equals the current population, N0 represents the initial population, r represents the rate of growth, and t represents the time in appropriate units. Use
the provided information to determine the rate of growth of the mosquito population: 300,000 = 500,000
× r5
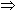 0.6 = r5
0.6 = r5
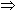 r ≈ 0.903 (the population is decreasing at about 10% per year). Use this r value
to solve the problem: N = 500,000 × (0.903)7
r ≈ 0.903 (the population is decreasing at about 10% per year). Use this r value
to solve the problem: N = 500,000 × (0.903)7
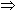 N ≈ 244,785 ≈ 245,000.
Correct Response: A. (Objective 0006) The situation can be modeled using an exponential
function of the form N equals N sub zero multiplied by r superscript t, where
N equals the current population, N subscript zero represents the initial population,
r represents the rate of growth, and t represents the time in appropriate units. Use
the provided information to determine the rate of growth of the mosquito population: Three hundred thousand
equals five hundred thousand times r superscript five leads to zero point six equals r superscript
five leads to r is almost equal to zero point nine zero three (the population is decreasing
at about ten percent per year). Use this r value to solve the problem: N equals five
hundred thousand multiplied by open parenthesis zero point nine zero three close parenthesis superscript
seven leads to N which is approximately two hundred and forty four thousand seven hundred and
eighty five which is approximately two hundred and forty five thousand.
N ≈ 244,785 ≈ 245,000.
Correct Response: A. (Objective 0006) The situation can be modeled using an exponential
function of the form N equals N sub zero multiplied by r superscript t, where
N equals the current population, N subscript zero represents the initial population,
r represents the rate of growth, and t represents the time in appropriate units. Use
the provided information to determine the rate of growth of the mosquito population: Three hundred thousand
equals five hundred thousand times r superscript five leads to zero point six equals r superscript
five leads to r is almost equal to zero point nine zero three (the population is decreasing
at about ten percent per year). Use this r value to solve the problem: N equals five
hundred thousand multiplied by open parenthesis zero point nine zero three close parenthesis superscript
seven leads to N which is approximately two hundred and forty four thousand seven hundred and
eighty five which is approximately two hundred and forty five thousand.
Question 33
33. A line L is represented by the equation 2x − 3y + 12 = 0. Which
of the following equations represents a line that is parallel to line L and intersects the
x-axis when x = 6?
-
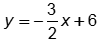
-
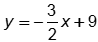
-
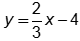
-
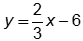
Answer to question 33
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: C. (Objective 0006) Solve 2x −
3y + 12 = 0 for y to get:
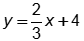 .
The slope of line L is
.
The slope of line L is
 . Parallel lines have
the same slope, so the slope needed is also
. Parallel lines have
the same slope, so the slope needed is also
 . Since the parallel
line intersects the x-axis when x = 6, this line passes through the point (6, 0).
Using the point-slope form of a linear equation,
. Since the parallel
line intersects the x-axis when x = 6, this line passes through the point (6, 0).
Using the point-slope form of a linear equation,
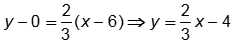 .
Correct Response: C. Solve two x minus three y plus twelve equals zero for y to
get: y equals two thirds x plus four. The slope of line L is two thirds. Parallel lines have the same
slope, so the slope needed is also two thirds. Since the parallel line intersects the x-axis when x
equals six, this line passes through the point six comma zero. Using the point-slope form of a linear
equation, y minus zero equals two thirds open parenthesis x minus six end parenthesis, which becomes
y equals two thirds x minus four.
.
Correct Response: C. Solve two x minus three y plus twelve equals zero for y to
get: y equals two thirds x plus four. The slope of line L is two thirds. Parallel lines have the same
slope, so the slope needed is also two thirds. Since the parallel line intersects the x-axis when x
equals six, this line passes through the point six comma zero. Using the point-slope form of a linear
equation, y minus zero equals two thirds open parenthesis x minus six end parenthesis, which becomes
y equals two thirds x minus four.
Question 34
34. The tendency of graphing calculators to inappropriately connect adjacent points would pose a problem
when a student is analyzing a function with which of the following characteristics?
- local maximums
- points of inflection
- horizontal asymptotes
- vertical asymptotes
Answer to question 34
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0006) When using certain
windows or zooms, the "draw" command written into calculator software will connect points without regard
for discontinuity of functions. For example, if
 ,
the zoom standard shows the result below. Students interpret the almost vertical piece connecting the
two curves as part of the graph when the graph is actually disjoint.
,
the zoom standard shows the result below. Students interpret the almost vertical piece connecting the
two curves as part of the graph when the graph is actually disjoint.
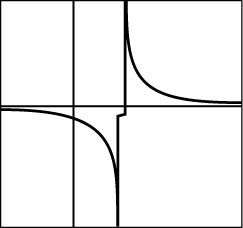 Correct Response: D. (Objective 0006) When using certain windows or zooms, the
draw command written into calculator software will connect points without regard for discontinuity of
functions. For example, if f of x equals one over the quantity x minus two, the zoom standard shows
the result below. Students interpret the almost vertical piece connecting the two curves as part of
the graph when the graph is actually disjoint.
Correct Response: D. (Objective 0006) When using certain windows or zooms, the
draw command written into calculator software will connect points without regard for discontinuity of
functions. For example, if f of x equals one over the quantity x minus two, the zoom standard shows
the result below. Students interpret the almost vertical piece connecting the two curves as part of
the graph when the graph is actually disjoint.
The display of a graphing calculator of the graph of the function f of x equals one over the quantity
x minus two is shown on the coordinate plane. That is, as the graph of f of x approaches negative infinity,
the graph lies predominantly in the third quadrant and has a horizontal asymptote at y equals zero;
as the graph approaches the vertical asymptote from the negative direction, there appears to be a small
horizontal line as it crosses the vertical asymptote x equals two; and, as the graph approaches positive
infinity, it lies completely in the first quadrant and has a horizontal asymptote at y equals zero.
Question 35
35. Which of the following descriptions is represented by the slope of the tangent line
 in the graph below?
in the graph below?
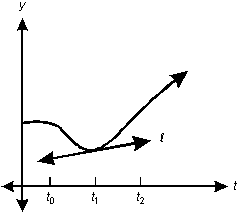
There is a coordinate plane on which the vertical axis is labeled y and the horizontal axis is labeled
t. The horizontal axis is marked with three distinct values from left to right: t subscript zero, t
subscript one, and t subscript two. The graphs of two functions are shown on the plane: the tangent
line l and the curve to which line l is tangent. The point of tangency is a local minimum of the curve.
The t coordinate of this minimum point is t subscript one.
- the average rate at which t is changing with respect to y
between t0 and t2the average rate
at which t is changing with respect to y between t subscript zero and t subscript two
- the average rate at which y is changing with respect to t
between t0 and t2the average rate
at which y is changing with respect to t between t subscript zero and t subscript two
- the instantaneous rate at which t is changing with respect to
y at t1 the instantaneous rate at which t is changing
with respect to y at t subscript one
- the instantaneous rate at which y is changing with respect to
t at t1 the instantaneous rate at which y is changing
with respect to t at t subscript one
Answer to question 35
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0007) The average rate of
change of this function over the interval from t0 to t2 is the
change in y divided by the change in t. This describes the slope of a segment connecting
two points on the curve. The definition of the derivative is the limit of the average rate of change
as the time interval approaches zero. If the distances between t0 and t1,
as well as t1 and t2 approach zero, the slopes of the associated
segments approach the slope of the tangent line at t1 and the associated average
rates of change approach the instantaneous rate of change at t1.
Correct Response: D. (Objective 0007) The average rate of change of this function
over the interval from t subscript zero to t subscript two is the change in y divided by the change
in t. This describes the slope of a segment connecting two points on the curve. The definition of the
derivative is the limit of the average rate of change as the time interval approaches zero. If the distances
between t subscript zero and t subscript one, as well as t subscript one and t subscript two approach
zero, the slopes of the associated segments approach the slope of the tangent line at t subscript one
and the associated average rates of change approach the instantaneous rate of change at t subscript
one.
Question 36
36. If
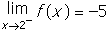 and
and
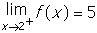 ,
then the limit of f(x) as x approaches 2:
,
then the limit of f(x) as x approaches 2:
- is zero.
- is two.
- is infinity.
- does not exist.
Answer to question 36
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0007) The value of f(x)
approaches −5 when x approaches 2 from the left (negative) side, and the value of f(x)
approaches 5 when x approaches 2 from the right (positive) side. When the limits of a function
as x approaches a point from the left and the right are not equal, the limit of the function
as x approaches the point does not exist.
Correct Response: D. (Objective 0007) The value of f of x approaches negative
five when x approaches two from the left (negative) side, and the value of f of x approaches
five when x approaches two from the right (positive) side. When the limits of a function as
x approaches a point from the left and the right are not equal, the limit of the function as
x approaches the point does not exist.
Question 37
37. If cos(x + y) = cos x cos y − sin x sin ycosine(x plus y) equals cosine x cosine y minus sine x sine y, which of the following expressions simplifies to cos(–x + y)cosine(negative x plus y)?
- –cos x cos y − sin x sin ynegative cosine x cosine y minus sine x sin y
- cos x cos y − sin x sin y cosine x cosine y minus sine x sine y
- –cos x cos y + sin x sin y negative cosine x cosine y plus sine x sine y
- cos x cos y + sin x sin y cosine x cosine y plus sine x sine y
Answer to question 37
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0007) Replace x in the given equation with –x: cos(–x + y) = cos –x cos y − sin –x sin y. Then apply the trigonometric identities cos –x = cos x and sin –x = –sin x to simplify the expression.
This yields the result cos(–x + y) = cos x cos y + sin x sin y.
Correct Response: B. (Objective 0007) Replace x in the given equation with negative x: cosine(negative x plus y) equals cosine negative x cosine y minus sine negative x sine y. Then apply the trigonometric identities cosine negative x equals cosine x and sine negative x equals negative sine x to simplify the expression. This yields the result cosine open parenthesis negative x plus y close parenthesis equals cosine x cosine y plus sine x sine y
Question 38
38. The diagram below shows the graph of f(x) = x3 + 3x2
+ 3. The point P has coordinates (−1, 5). What is the limit of the slope of the line
through points P and Q as Q approaches P?
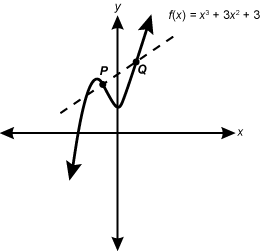
f of x equals x cubed plus three x squared plus three continuous graph starts in the third quadrant
and increase past the x axis into the second quadrant where it peaks then starts to decrease to point
p at open parenthesis negative one comma five close parenthesis decreases to the y axis where it starts
to increase into the first quadrant at a steady pace to point q that has some x value and a higher y
value then point p. there is a dashed line drawn through point p and point q that is decreasing from
point q to point p.
- −3negative 3
- −2negative 2
- 0
- 1
Answer to question 38
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: A. (Objective 0007) As Q approaches
P, the average rate at which y changes with respect to x between P
and Q,
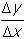 , approaches the
instantaneous rate at which y changes with respect to x at point P, i.e.,
the slope of f(x) at P. This is the definition of the derivative, and the
limit of the slope of the line through points P and Q as Q approaches P
can be calculated as the derivative of f (x) evaluated at point P: f′(x)
= 3x2 + 6x
, approaches the
instantaneous rate at which y changes with respect to x at point P, i.e.,
the slope of f(x) at P. This is the definition of the derivative, and the
limit of the slope of the line through points P and Q as Q approaches P
can be calculated as the derivative of f (x) evaluated at point P: f′(x)
= 3x2 + 6x
 f′(−1) = 3(−1)2 + 6(−1) = −3.
Correct Response: A. (Objective 0007) As Q approaches P, the
average rate at which y changes with respect to x between P and Q,
delta y over delta x, approaches the instantaneous rate at which y changes with respect to
x at point P, i.e., the slope of f of x at P. This is the definition of the
derivative, and the limit of the slope of the line through points P and Q as Q
approaches P can be calculated as the derivative of f of x evaluated at point P: f prime of
x equals three x squared plus six x leads to f prime of negative one equals three open parenthesis negative
one close parenthesis squared plus six open parenthesis negative one close parenthesis equals negative three.
f′(−1) = 3(−1)2 + 6(−1) = −3.
Correct Response: A. (Objective 0007) As Q approaches P, the
average rate at which y changes with respect to x between P and Q,
delta y over delta x, approaches the instantaneous rate at which y changes with respect to
x at point P, i.e., the slope of f of x at P. This is the definition of the
derivative, and the limit of the slope of the line through points P and Q as Q
approaches P can be calculated as the derivative of f of x evaluated at point P: f prime of
x equals three x squared plus six x leads to f prime of negative one equals three open parenthesis negative
one close parenthesis squared plus six open parenthesis negative one close parenthesis equals negative three.
Question 39
39. The shaded area under the curve y = −negative
x2 squared
+ 9 can be estimated by finding the area of each of the shaded regions shown and summing them using
the expression
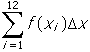 , where Δx represents the width
of each rectangle.
, where Δx represents the width
of each rectangle.
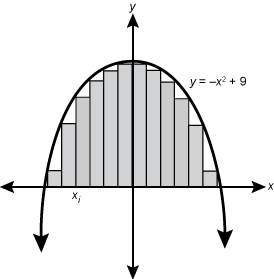
opened down of y equals negative x squared plus nine. the vertex is at the point where X is zero and
y is some positive value and the graph decreases to the left through the second quadrant slowly at first
then decreases more quickly passing the negative x axis and continuing to decrease through the third
quadrant. the graph decreases from the vertex to the right in the first quadrant slowly at first then
more quickly as it passed the positive x axis into the fourth quadrant. there are twelve shaded rectangles
drawn from the x axis up to the curve of the parabola where the top left corner is on the parabola at
some y value and the width of each rectangle is delta x.
What is the exact value of the area under the curve?
- 9
- 18
- 27
- 36
Answer to question 39
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: D. (Objective 0007) The exact area under
the curve can be calculated as the limit of the sum of the shaded rectangles as the number of rectangles
increases to infinity, i.e., as Δx decreases toward zero. This is calculated as the definite
integral of the curve y = −x2 + 9, −3 ≤ x ≤
3. Thus:
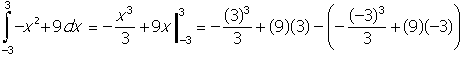 = −9 + 27 − (9 − 27) = 36.
Correct Response: D. (Objective 0007) The exact area under the curve can be calculated
as the limit of the sum of the shaded rectangles as the number of rectangles increases to infinity,
i.e., as delta x decreases toward zero. This is calculated as the definite integral of the
curve y equals negative x squared plus nine comma negative three less than or equal to x less than or
equal to three. Thus: integral from negative three to three of negative x squared plus nine d x equals
negative x cubed over three baseline plus nine x over interval negative three to three equals negative
three cubed over three plus open parenthesis nine close parenthesis open parenthesis three close parenthesis
minus open parenthesis negative negative three cubed over three baseline plus open parenthesis nine
close parenthesis open parenthesis negative three close parenthesis close parenthesis equals negative
nine plus twenty seven minus open parenthesis nine minus twenty seven close parenthesis equals thirty
six.
= −9 + 27 − (9 − 27) = 36.
Correct Response: D. (Objective 0007) The exact area under the curve can be calculated
as the limit of the sum of the shaded rectangles as the number of rectangles increases to infinity,
i.e., as delta x decreases toward zero. This is calculated as the definite integral of the
curve y equals negative x squared plus nine comma negative three less than or equal to x less than or
equal to three. Thus: integral from negative three to three of negative x squared plus nine d x equals
negative x cubed over three baseline plus nine x over interval negative three to three equals negative
three cubed over three plus open parenthesis nine close parenthesis open parenthesis three close parenthesis
minus open parenthesis negative negative three cubed over three baseline plus open parenthesis nine
close parenthesis open parenthesis negative three close parenthesis close parenthesis equals negative
nine plus twenty seven minus open parenthesis nine minus twenty seven close parenthesis equals thirty
six.
Question 40
40. The mosquito population in a particular area is increasing at a rate of 15,000(t3
+ 1) mosquitoes per day on day t. If the initial population on day t = 0 is estimated
to be 3,000 mosquitoes and assuming none will die, how many mosquitoes will there be after 2 days?
- 63,000
- 93,000
- 138,000
- 168,000
Answer to question 40
- Answer Enter to expand or collapse answer. Answer expanded
- Correct Response: B. (Objective 0007) Since the rate of growth,
or rate of change, is 15,000(t3 + 1) mosquitoes per day on day t,
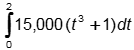 calculates the total number of mosquitoes by which the population will grow after 2 days.
calculates the total number of mosquitoes by which the population will grow after 2 days.
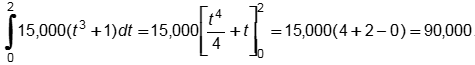 .
.
Because there were already 3,000 mosquitoes, so the population after 2 days will be 93,000.
Correct Response: B. (Objective 0007) Since the rate of growth, or rate of change,
is fifteen thousand open parenthesis t cubed plus one close parenthesis mosquitoes per day on day t,
the integral from zero to two of fifteen thousand open parenthesis t cubed plus one close parenthesis
d t calculates the total number of mosquitoes by which the population will grow after two days. The
integral from zero to two of fifteen thousand open parenthesis t cubed plus one close parenthesis d
t equals fifteen thousand open bracket one fourth t to the fourth power plus t close bracket, evaluated
at two and zero, equals fifteen thousand open parenthesis four plus two minus zero close parenthesis
equals ninety thousand. Because there were already three thousand mosquitoes, so the population after two
days will be ninety three thousand.