Mathematics (Grades 5–12)

Subtest 2 Sample Items

Recommendation for individuals using a screenreader: please set your punctuation settings to "most."

Expand All | Collapse All

Question 1

1. A 26 ft. clothesline reaches across a city alley from a 5th floor window in one building to a 4th floor window in another building. If the vertical drop between the windows is 10 ft., what is the approximate angle of elevation, θ, of the clothesline?1. A 26 foot clothesline reaches across a city alley from a fifth floor window in one building to a fourth floor window in another building. If the vertical drop between the windows is 10 feet, what is the approximate angle of elevation, theta, of the clothesline?

1. 21°21 degrees
2. 23°23 degrees
3. 67°67 degrees
4. 69°69 degrees

Answer to question 1

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0008) The situation can be represented by a right triangle with one leg parallel to the ground, the other leg equal to the vertical drop from the 5th floor window to the 4th floor window, and the hypotenuse equal to the length of the clothesline. The angle of elevation is the angle between the leg parallel to the ground and the hypotenuse. Using the sine ratio, 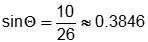and using inverse sine, sin^–1(0.3846) ≈ 23°. Correct Response: B. (Objective 0008) The situation can be represented by a right triangle with one leg parallel to the ground, the other leg equal to the vertical drop from the 5th floor window to the 4th floor window, and the hypotenuse equal to the length of the clothesline. The angle of elevation is the angle between the leg parallel to the ground and the hypotenuse. Using the sine ratio, sin of theta equals ten twenty sixths which approximately equals zero point three eight four six and using inverse sine, sine superscript negative 1 ( 0 point 3846 ) almost equal to 23 degrees.

Question 2

2. A human body that weighs 160 pounds contains 5 liters of blood. If the density of blood is 1060 kg/m³kiligrams per meter cubed, one liter is 0.001 m³0 point 001 meter cubed, and one kilogram weighs approximately 2.2 pounds, approximately what percentage of the body weight is blood?

1. 1.5 %1 point 5 percent
2. 7.3 %7 point 3 percent
3. 45 %45 percent
4. 72 %72 percent

Answer to question 2

Answer Enter to expand or collapse answer. Answer expanded

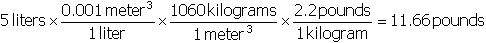
Correct Response: B. (Objective 0008) Correct Response: B. (Objective 0008) five liters times start fraction numerator zero point zero zero one meters cubed denominator one liter end fraction times start fraction one thousand sixty kilograms denominator one meters cubed end fraction times start fraction numerator two point two pounds denominator one kilogram end fraction equals eleven point six six pounds start fraction numerator eleven point six six pounds denominator one hundred and sixty pounds end fraction equals zero point zero seven two eight seven five or approximately seven point three percent

Question 3

3. A ladder leaning against a wall makes a 20° degree angle with the ground. When the bottom of the ladder is moved 2 ft. closer to the wall, the ladder makes a 40° degree angle with the ground. Approximately how long is the ladder?

1. 8.5 ft.
2. 10 ft.
3. 11.5 ft.
4. 13 ft.

Answer to question 3

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0008) This problem can be represented by a diagram, as shown below.

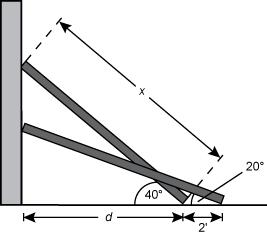
In the diagram, x represents the length of the ladder, and d represents the distance of the base of the ladder from the wall, after it is moved. Therefore, 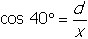and 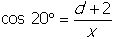, so x cos 40° = d and x cos 20° = d + 2. Then, x (cos 40° − cos 20°) = d − (d + 2) = −2 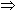x(−0.173648) = −2 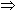x ≈ 11.5 ft. Correct Response: C. (Objective 0008) This problem can be represented by a diagram, as shown below.
A ladder of length x leans against a vertical wall forming a twenty degree angle between the ladder and the floor. The base of the ladder is a distance of d plus two feet from the wall. The ladder is moved two feet closer to the wall to a distance of d where it now makes a forty degree angle between the ladder and the floor.
In the diagram, x represents the length of the ladder, and d represents the distance of the base of the ladder from the wall after it is moved. Therefore, cosine forty degrees equals d over x and cosine twenty degrees equals start fraction numerator d plus two denominator x end fraction, so x cosine forty degrees equals d and x cosine twenty degrees equals d plus two. Then, x open parenthesis cosine forty degrees minus cosine twenty degrees close parenthesis equals d minus open parenthesis d plus two close parenthesis equals negative two leads to x open parenthesis negative zero point one seven three six four eight close parenthesis equals negative two leads to x is approximately eleven point five feet.

Question 4

4. A balloon has the shape of a right cylinder of length L, with a hemisphere of radius r on each end, as shown in the diagram below.

The length of the cylindrical part of the balloon, L, is 8 times the radius, r. Air is added to the balloon, doubling the surface area while maintaining a similar shape. Which of the following expressions represents the length of the larger balloon?

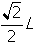1. start fraction square root of 2 end root over two end fraction L
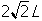2. 2 times square root of 2 end root L
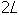3. 2L
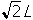4. square root of 2 end root L

Answer to question 4

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0008) The surface area of the shape can be expressed as the sum of the surface areas of the two hemispheres (which is equivalent to one sphere) plus the surface area of the cylinder (without its bases): 4πr² + 2πrL = 4πr² + 2πr(8r) = 4πr² + 16πr² = 20πr². Since the enlarged balloon is similar in shape to the original balloon, the length of the enlarged balloon (L_E) is eight times its radius: L_E = 8r_E. The surface area of the enlarged balloon is double that of the original balloon, or 2 × 20πr² = 40πr²; thus, the equation for the surface area of the enlarged balloon can be written as area = 4πr_E² + 2πr_E(8r_E) = 40πr². This implies that 20πr_E² = 40πr² ⇒ r_E² = 2r² ⇒ r_E = r√ 2  ⇒ 8r_E = 8r√ 2  = √ 2 L. Correct Response: D. (Objective 0008) The surface area of the shape can be expressed as the sum of the surface areas of the two hemispheres (which is equivalent to one sphere) plus the surface area of the cylinder (without its bases): 4 pi r squared plus 2pi rL equals 4pi r squared plus 2pi r left paren 8r right paren equals 4pi r squared plus 16pi rsquared equals 20pi rsquared. Since the enlarged balloon is similar in shape to the original balloon, the length of the enlarged balloon left paren L_E right paren is eight times its radius: L_E equals 8r_E. The surface area of the enlarged balloon is double that of the original balloon, or 2 times 20pi r squared = 40pi r squared; thus, the equation for the surface area of the enlarged balloon can be written as area = 4pi r_E squared plus 2pi r_E left paren 8r_E right paren equals 40pi r squared. This implies that 20pi r_Esquared equals 40pi rsquared which becomes r_E squared equals 2r squared which becomes r_E equals r square root of 2 which becomes 8r_E equals 8r square root of 2 equals square root of 2L

Question 5

5. What is the approximate area of the shaded semicircular arch shown below?

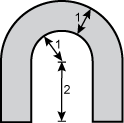
the inner border of the arch has a radius of one unit. the thickness of the arch from the inner border to the outer border is also one unit.

1. 5.6 square units
2. 6.7 square units
3. 7.1 square units
4. 8.7 square units

Answer to question 5

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0008) The area of the arch can be calculated as the sum of the areas of two rectangles plus the difference between half the area of a large circle and half the area of a small circle: 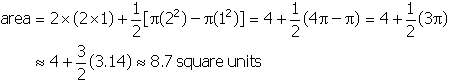Correct Response: D. (Objective 0008) The area of the arch can be calculated as the sum of the areas of two rectangles plus the difference between half the area of a large circle and half the area of a small circle: area equals two times open parenthesis two times one close parenthesis plus one half left bracket pi open parenthesis two squared close parenthesis minus pi open parenthesis one squared close parenthesis right bracket equals four plus one half open parenthesis four pi minus pi close parenthesis equals four plus one half open parenthesis three pi close parenthesis approximately equal to four plus three halves open parenthesis three point one four close parenthesis approximately equal to eight point seven square units.

Question 6

6. A spherical ornament is packed in a box shaped like a cube. The interior dimensions of the box are each equal to the diameter of the sphere. The spherical ornament takes up approximately what percent of the space in the box?

1. 52%
2. 61%
3. 70%
4. 79%

Answer to question 6

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0008) The volume of a sphere with radius r is 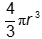. Since the interior dimensions of the box are each equal to the diameter of the sphere, the volume of the space in the box is (2r)³ or 8r³. The ratio of the volumes is 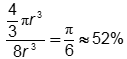. Correct Response: A. (Objective 0008) The volume of a sphere with radius r is four thirds pi r cubed. Since the interior dimensions of the box are each equal to the diameter of the sphere, the volume of the space in the box is open parenthesis two r close parenthesis cubed or eight r cubed. The ratio of the volumes is the quantity four thirds pi r cubed over the quantity eight r cubed which equals one sixth pi which approximately equals fifty two percent.

Question 7

7. If the measure of one of the interior angles of regular polygon P is three times the measure of one of the exterior angles of a 9-sided regular polygon, how many sides does polygon P have?

1. 6
2. 8
3. 10
4. 12

Answer to question 7

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0009) The measure of an interior angle of a regular polygon with n sides can be expressed as 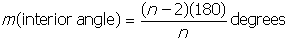. The measure of an exterior angle of a polygon with n sides can be expressed as the supplement of this, i.e., 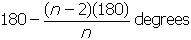. Thus, the number of sides in polygon P can be found by solving the equation 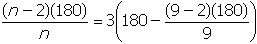. So, 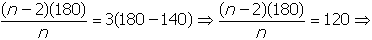(n − 2)(180) = 120n. This implies that 60n = 360, and n = 6. Correct Response: A. (Objective 0009) The measure of an interior angle of a regular polygon with n sides can be expressed as m open parenthesis interior angle close parenthesis equals start fraction numerator open parenthesis n minus two close parenthesis open parenthesis one hundred and eighty close parenthesis denominator n end fraction degrees. The measure of an exterior angle of a polygon with n sides can be expressed as the supplement of this, i.e., one hundred and eighty minus start fraction numerator open parenthesis n minus two close parenthesis open parenthesis one hundred and eighty close parenthesis denominator n end fraction degrees. Thus, the number of sides in polygon P can be found by solving the equation start fraction numerator open parenthesis n minus two close parenthesis open parenthesis one hundred and eighty close parenthesis denominator n end fraction equals three open parenthesis one hundred and eighty minus start fraction numerator open parenthesis nine minus two close parenthesis open parenthesis one hundred and eighty close parenthesis denominator nine end fraction close parenthesis.
So, start fraction numerator open parenthesis n minus two close parenthesis open parenthesis one hundred and eighty close parenthesis denominator n end fraction equals three open parenthesis one hundred and eighty minus one hundred and forty close parenthesis leads to start fraction numerator open parenthesis n minus two close parenthesis open parenthesis one hundred and eighty close parenthesis denominator n end fraction equals one hundred and twenty leads to open parenthesis n minus two close parenthesis open parenthesis one hundred and eighty close parenthesis equals one hundred and twenty n. This implies that sixty n equals three hundred and sixty, and n equals six.

Question 8

8. A cylindrical hole 2 ft. in diameter is drilled straight into the ground to make a well. If the dirt removed from the hole forms a cone-shaped pile with a height of 6 ft. and a diameter of 10 ft. at the base, how deep is the well?

1. 12 ft.
2. 50 ft.
3. 174 ft.
4. 200 ft.

Answer to question 8

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0009) The volume of the cylindrical hole can be calculated as V_cyl = πr²h = π(1)d, where d represents the depth of the hole. The volume of the conical dirt pile can be calculated as 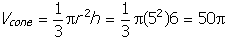. Since V_cyl = V_cone, πd = 50π, and d = 50 ft. Correct Response: B. (Objective 0009) The volume of the cylindrical hole can be calculated as volume of cylinder equals pi r squared h equals pi open parenthesis one close parenthesis d, where d represents the depth of the hole. The volume of the conical dirt pile can be calculated as v of cone equals one third pi r squared h equals one third pi open parenthesis five squared close parenthesis six equals fifty pi. Since volume of a cylinder equals volume of a cone comma pi d equals fifty pi and d equals fifty feet.

Question 9

9. If 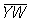is a median and an altitude of Δtriangle XYZ, then Δtriangle XYZ must be:

1. equilateral.
2. acute.
3. isosceles.
4. obtuse.

Answer to question 9

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0009) A median of a triangle is a line segment from a vertex that intersects and bisects the opposite side. An altitude of a triangle is a line segment from a vertex that is perpendicular to the opposite side. If the line segment from a vertex both bisects and is perpendicular to the opposite side, the triangle may be equilateral, but it must be isosceles. Correct Response: C. (Objective 0009) A median of a triangle is a line segment from a vertex that intersects and bisects the opposite side. An altitude of a triangle is a line segment from a vertex that is perpendicular to the opposite side. If the line segment from a vertex both bisects and is perpendicular to the opposite side, the triangle may be equilateral, but it must be isosceles.

Question 10

10. Use the construction described below to answer the question that follows.

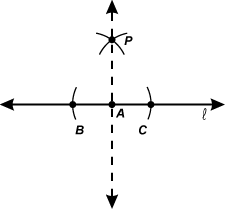
To construct a line perpendicular to a given line ℓ at point A: 1. From point A, construct arcs with the same radius intersecting line ℓ at points B and C. 2. From each of points B and C, construct arcs with the same radius intersecting at point P. 3. Draw line PAP A.

To prove that the construction shown results in line PA perpendicular to line ℓ, which of the following congruent triangle theorems should be used?

1. ASAA S A
2. SSSS S S
3. AASA A S
4. HL H L

Answer to question 10

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0009) By construction, AB ≅ AC and PB ≅ PC. PC is congruent to itself by the reflexive property. △PBA ≅ △PCA by SSS. Correct Response: B. (Objective 0009) By construction, segment A B is congruent to segment A C and segment PB is congruent to segment PC. Segment P A is congruent to itself by the reflexive property. Triangle P B A is congruent to triangle P C A by S S S.

Question 11

11. The graphs of the polar equation r = 2cosθ and the rectangular equation x² + y² = 4 intersect at a point on the:

11. The graphs of the polar equation r equals two cosine theta and the rectangular equation x squared plus y squared equals four intersect at a point on the:

1. positive x-axis.
2. negative x-axis.
3. positive y-axis.
4. negative y-axis.

Answer to question 11

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0010) The equation x² + y² = 4 represents a circle centered at the origin with a radius of 2 units, so this circle intersects the axes at (2, 0), (0, 2), (–2, 0), and (0, –2). Thus, in the equation r = 2cosθ, either r = 2 or r = –2. If r = 2, cosθ = 1 and θ = 0° or 360°, so the graph intersects the x-axis at (2, 0). If r = –2, cosθ = –1 and θ = 180°. Negative radii reflect points over the origin, so the graph again intersects the x-axis at (2, 0). Thus the two graphs intersect on the positive x-axis. Correct Response: A. (Objective 0010) The equation x squared plus y squared equals four represents a circle centered at the origin with a radius of two units, so this circle intersects the axes at ( 2 comma 0 ), ( 0 comma 2 ), ( negative 2 comma 0 ), and ( 0 comma negative 2 ). Thus, in the equation r equals 2 cosine theta, either r equals 2 or r equals negative 2. If r equals 2, cosine theta equals 1 and theta equals 0 degrees or three hundred sixty degrees, so the graph intersects the x-axis at ( 2 comma 0 ). If r equals negative 2, cosine theta equals negative 1 and theta equals one hundred eighty degrees. Negative radii reflect points over the origin, so the graph again intersects the x-axis at ( 2 comma 0 ). Thus the two graphs intersect on the positive x-axis.

Question 12

12. Circle A in the coordinate plane has a diameter with coordinates (−negative 1, 7) and (7, 7). Circle B has a diameter with coordinates (a, −negative 2) and (3, −negative 2). If circle A has an area that is 16 times that of circle B, what are the possible values of a?

1. 2 or −negative 2
2. 3 or 5
3. 1 or −negative 1
4. 1 or 5

Answer to question 12

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0010) The diameter of circle A is 7 − (–1) = 8, so its radius is 4 units and its area is π(4²) = 16π. Therefore, the area of circle B must be π, and circle B must have a radius of 1. If the endpoint of one diameter of circle B is at (3, −2) and the radius of B is 1 with a y-coordinate of −2, the other endpoint must have the coordinates (1, −2) or (5, −2). Correct Response: D. (Objective 0010) The diameter of circle A is 7 minus open parenthesis negative 1 close parenthesis equals eight, so its radius is four units and its area is pi open parenthesis four square close parenthesis equals sixteen pi. Therefore, the area of circle B must be pi, and circle B must have a radius of one. If the endpoint of one diameter of circle B is at open parenthesis three comma negative two close parenthesis and the radius of B is one with a y-coordinate of negative two, the other endpoint must have the coordinates open parenthesis one comma negative two close parenthesis or open parenthesis five comma negative two close parenthesis.

Question 13

13. On a set of three-dimensional coordinate axes, a cube is located with its base coordinates at (0, 0, 0), (2, 0, 0), (0, 2, 0), and (2, 2, 0). The diagonals of the cube intersect at point M. What is the distance of point M to a vertex of the cube?

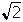1. square root of 2
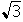2. square root of 3
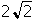3. 2 times the square root of 2
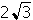4. 2 times the square root of 3

Answer to question 13

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0010) One of the diagonals has as endpoints the coordinates (2, 0, 0) and (0, 2, 2). Using the distance formula, the length of this diagonal is 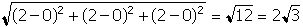. Point M is the midpoint of the diagonal, so the distance of M to a vertex is . Correct Response: B. (Objective 0010) One of the diagonals has as endpoints the coordinates open parenthesis two comma zero comma zero close parenthesis and open parenthesis zero comma two comma two close parenthesis. Using the distance formula, the length of this diagonal is "start square root open parenthesis two minus zero close parenthesis squared plus open parenthesis two minus zero close parenthesis squared plus open parenthesis two minus zero close parenthesis squared end square root equals square root of twelve equals two times the square root of three. Point M is the midpoint of the diagonal, so the distance of M to a vertex is one half times two times the square root of three equals the square root of three.

Question 14

14. Which of the following transformations reflects a figure in the coordinate plane over the line y = x?

1. (x, y) →right arrow (y, x)
2. (x, y) →right arrow (y, − negative x)
3. (x, y) →right arrow (x, − negative y)
4. (x, y) →right arrow (− negative x, − negative y)

Answer to question 14

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0010) The transformation (x, y) → (y, x) interchanges the x- and y-coordinates, resulting in the reflection of a point across the line y = x. Correct Response: A. (Objective 0010) The transformation open parenthesis x comma y close parenthesis right arrow open parenthesis y comma x close parenthesis interchanges the x- and y-coordinates, resulting in the reflection of a point across the line y equals x.

Question 15

15. Use the triangle below to answer the question that follows.

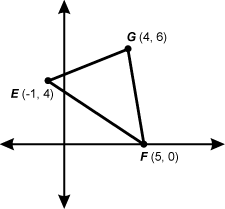
What is the equation of the perpendicular bisector of side EF E F of the triangle shown?

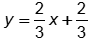
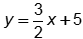
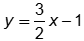
Answer to question 15

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0010) The perpendicular bisector of side EF must go through the midpoint of side EF. Calculate the midpoint to get: (2, 2). The slope of side EF is 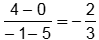. The slope of a line perpendicular to side EF must be the negative reciprocal of 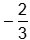, or 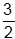. Use the point-slope form of the equation of a line to get: 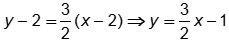. Correct Response: D. (Objective 0010) The perpendicular bisector of side E F must go through the midpoint of side E F. Calculate the midpoint to get the quantity five plus negative one over two comma the quantity zero plus four over two which becomes two comma two. The slope of side E F is the quantity four minus zero over the quantity negative one minus five which equals negative two thirds. The slope of a line perpendicular to side E F must be the negative reciprocal of negative two thirds or three halves. Use the point-slope form of the equation of a line to get y minus two equals three halves the quantity open parenthesis x minus two close parenthesis which yields y equals three halves x minus one.

Question 16

16. Construction of a box plot to display data is particularly useful when:

1. the number of data points is relatively small.
2. a quick preliminary look at the shape of the data distribution is needed.
3. comparisons are to be made between data sets of different sizes.
4. identification of individual data points is desirable.

Answer to question 16

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0011) The advantages of box plots are: the box plot highlights the important features of the data; the box plot makes it easier to focus on the median, quartiles, and extreme values; and the box plot makes it easier to compare these features to the same features in another data set by putting them on the same set of axes. Correct Response: C. (Objective 0011) The advantages of box plots are: the box plot highlights the important features of the data; the box plot makes it easier to focus on the median, quartiles, and extreme values; and the box plot makes it easier to compare these features to the same features in another data set by putting them on the same set of axes.

Question 17

17. Which of the following formats most clearly shows the overall shape and symmetry of a univariate data distribution?

1. histogram
2. circle graph
3. scatter plot
4. line graph

Answer to question 17

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0011) A histogram consists of a horizontal scale for data values, a vertical scale for frequencies, and bars that represent the various data classes. Together the bars provide an effective visual representation of the shape and symmetry of a univariate data distribution. A circle graph is an effective display of categorical data, a scatter plot effectively displays bivariate data, and a line graph effectively displays time-series-dependent data. Correct Response: A. (Objective 0011) A histogram consists of a horizontal scale for data values, a vertical scale for frequencies, and bars that represent the various data classes. Together the bars provide an effective visual representation of the shape and symmetry of a univariate data distribution. A circle graph is an effective display of categorical data, a scatter plot effectively displays bivariate data, and a line graph effectively displays time-series-dependent data.

Question 18

18. Which of the following statements describes the data in the stem-and-leaf plot below?

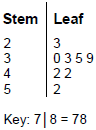
stem two leaf three. stem three leaf zero three five nine. stem four leaf two two. stem five leaf two. key: stem seven leaf eight equals seventy eight.

1. The mean and mode are equal and the median is smaller.
2. The mean is greater than the median and smaller than the mode.
3. The mean is less than the mode but greater than the median.
4. The mean and median are equal and the mode is larger.

Answer to question 18

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0011) Here the mean is calculated as . The median is the middle value, which in this case is the midpoint between 35 and 39, that is, 37. The mode is the most frequent value, 42. Thus, the mean and median are equal and the mode is larger. Correct Response: D. (Objective 0011) Here the mean is calculated as start fraction numerator twenty three plus thirty plus thirty three plus thirty five plus thirty nine plus forty two plus forty two plus fifty two denominator eight end fraction equals two hundred ninety six over eight equals thirty seven. The median is the middle value, which in this case is the midpoint between 35 and 39, that is, 37. The mode is the most frequent value, 42. Thus, the mean and median are equal and the mode is larger.

Question 19

19. The specifications for the composition of four candy mixtures are presented in the table below.

A quality control manager takes a sample of each mixture and finds the percentage of red jelly beans shown in the table. If the underlying distributions are assumed to be normally distributed, which mixture is most likely to concern the manager?

1. Mixture 1
2. Mixture 2
3. Mixture 3
4. Mixture 4

Answer to question 19

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0011) If the underlying distribution is normally distributed, approximately 95% of the samples taken should be within two standard deviations of the mean. In the sample from Mixture 3, 95% of the samples should occur within the interval (0.2, 0.36); the sample of 18% falls outside this interval, raising questions about the percentage of red jelly beans in Mixture 3. Correct Response: C. (Objective 0011) If the underlying distribution is normally distributed, approximately ninety five percent of the samples taken should be within two standard deviations of the mean. In the sample from Mixture 3, ninety five percent of the samples should occur within the interval open parenthesis zero point two comma zero point three six close parenthesis; the sample of eighteen percent falls outside this interval, raising questions about the percentage of red jelly beans in Mixture 3.

Question 20

20. Meteorologists sampled tropical cyclones and found that there is a strong tendency for cyclones to have greater wind speeds when the barometric pressure becomes very low as the cyclone approaches. A scatterplot of readings plots the lowest pressure reading on the horizontal axis and the maximum wind speed on the vertical axis for each cyclone in the sample. If the relationship is linear, which of the following values could represent the correlation coefficient for this sample?

1. 0.97
2. 0.42
3. –negative 0.36
4. –negative 0.88

Answer to question 20

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0011) The correlation coefficient, r, describes the strength of a linear relationship between two variables. The value of r lies between –1 and 1. The closer r is to –1 or 1, the better a linear equation fits the data. When the values of one variable decrease as the values of the other increase, then r is negative. Since the correlation between wind speed and barometric pressure is strong, and since wind speed increases as barometric pressure decreases, r must be close to –1. Thus, the best response is –0.88. Correct Response: D. (Objective 0011) The correlation coefficient, r, describes the strength of a linear relationship between two variables. The value of r lies between negative one and one. The closer r is to negative one or one, the better a linear equation fits the data. When the values of one variable decrease as the values of the other increase, then r is negative. Since the correlation between wind speed and barometric pressure is strong, and since wind speed increases as barometric pressure decreases, r must be close to negative one. Thus, the best response is negative zero point eight eight.

Question 21

21. If the two spinners below are spun simultaneously, what is the probability that the sum of the results will be greater than or equal to 7?

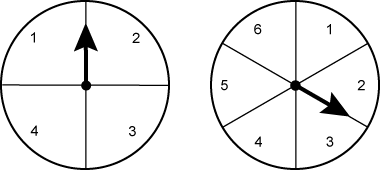
spinner on the left is divided into four equal sections labeled one, two, three, and four. Section one is top left section and continues clockwise with section two, three, and four. there is an arm with an arrow that is pointing up between section one and two on the spinner. spinner on the right is split into six equal sections labeled one, two, three, four, five, and six. the one section is just to the right of the middle top and and continues clockwise with sections two, three, four, five, and six. there is an arm with an arrow that is in between sections two and three.

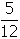
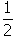
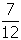
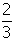
Answer to question 21

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0012) One method of solving this problem is to make a table that shows all the possible outcomes when the two spinners are spun. This is the sample space. The entry in each cell is the sum of the two spins.

Spinner Value	1	2	3	4	5	6
1	2	3	4	5	6	7
2	3	4	5	6	7	8
3	4	5	6	7	8	9
4	5	6	7	8	9	10

From the table, note that 10 of the 24 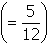equally likely results produce a sum greater than or equal to 7.

Question 22

22. The mean body length of beetles in a population is 7 mm. If body length in the population is normally distributed, and 95% of the beetles have a body length between 6.2 and 7.8 mm, what is the best approximation of the standard deviation of body length in the beetle population?

1. 0.2 mm
2. 0.4 mm
3. 0.6 mm
4. 0.8 mm

Answer to question 22

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0012) In a normally distributed population, approximately 95% of the data points will be within 2 standard deviations of the mean of the population. The interval within which the mean occurs here is 6.2–7.8, implying that the mean of the population is 7 ± 2(0.4), where 0.4 is the standard deviation. Correct Response: B. (Objective 0012) In a normally distributed population, approximately ninety five percent of the data points will be within 2 standard deviations of the mean of the population. The interval within which the mean occurs here is six point two through through seven point eight, implying that the mean of the population is seven plus or minus two open parenthesis zero point four close parenthesis, where zero point four is the standard deviation.

Question 23

23. A bag contains a large number of red and blue marbles. Fifty of the marbles are drawn randomly, of which 40 are red and 10 are blue. These are replaced in the bag, and then 3 more marbles are drawn. What is the approximate probability that exactly 2 of the 3 marbles drawn are red?

1. 64%
2. 51%
3. 38%
4. 13%

Answer to question 23

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0012) Since 40 of the 50 marbles drawn initially are red, the best estimate of the percentage of red marbles in the bag is 80%. When the 3 marbles are drawn, there are 3 possible outcomes that result in exactly 2 red marbles: RRB, RBR, and BRR. The approximate probability desired is the sum of the probabilities of these 3 possible outcomes: 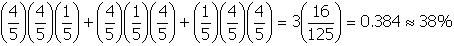. Correct Response: C. (Objective 0012) Since 40 of the 50 marbles drawn initially are red, the best estimate of the percentage of red marbles in the bag is eighty percent. When the 3 marbles are drawn, there are 3 possible outcomes that result in exactly 2 red marbles: R R B, R B R, and B R R. The approximate probability desired is the sum of the probabilities of these 3 possible outcomes: open parenthesis four fifths close parenthesis open parenthesis four fifths close parenthesis open parenthesis one fifth close parenthesis plus open parenthesis four fifths close parenthesis open parenthesis one fifth close parenthesis open parenthesis four fifths close parenthesis plus open parenthesis one fifth close parenthesis open parenthesis four fifths close parenthesis open parenthesis four fifths close parenthesis equals three open parenthesis sixteen over one hundred and twenty five close parenthesis equals zero point three eight four or approximately thirty eight percent.

Question 24

24. When designing surveys, the reason for using random sampling techniques rather than other techniques that may be more convenient is that random sampling will result in:

1. larger sample sizes.
2. smaller margins of error.
3. less potential bias.
4. higher levels of statistical significance.

Answer to question 24

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0012) Bias means that there are consistent, repeated deviations of the sample statistic from the parameter being measured. Larger sample sizes, smaller margins of error, and higher levels of statistical significance are all desirable in analyzing statistical data, but the underlying reason for insisting on random techniques is to reduce bias in the survey. Correct Response: C. (Objective 0012) Bias means that there are consistent, repeated deviations of the sample statistic from the parameter being measured. Larger sample sizes, smaller margins of error, and higher levels of statistical significance are all desirable in analyzing statistical data, but the underlying reason for insisting on random techniques is to reduce bias in the survey.

Question 25

25. There are four principal blood types: A, B, ABA B, and O. Approximately 40% of the U.S. population has Type A blood. On a particular day, a blood donation center needs 3 donors with Type A blood. Which of the following simulations should be used to estimate, on average, the number of donors who need to be seen in order to obtain exactly 3 donors with Type A blood?

1. Let heads on a coin represent Type A blood. Toss three coins and record the total number of heads. Repeat 100 times.
2. Let digits 0– through 3 represent Type A blood. Read single-digit random numbers until three digits with values below four are read. Record the number of random numbers read. Repeat 50 times.
3. On a ten-sided die, let 1, 2, 3, and 4 represent Type A blood. Roll three ten-sided dice and record the number of Type A results. Repeat 100 times.
4. Use a spinner with 4 equal sections, one for each of the 4 blood types. Count the number of spins taken until Type A has resulted three times. Repeat 50 times.

Answer to question 25

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0012) There are ten single digits, 0–9. Since 40% of the population has Type A blood, let the four digits 0, 1, 2, and 3 represent these people. Read random one-digit numbers from a random number generator until three digits representing Type A donors are found. The number of digits read in this trial represent the number of people seen until three Type A donors are found. Repeat 50 times and average the 50 results to get an estimate of the average number of people who need to be seen. Correct Response: B. (Objective 0012) There are ten single digits, zero through nine. Since forty percent of the population has Type A blood, let the four digits zero, one, two, and three represent these people. Read random one-digit numbers from a random number generator until three digits representing Type A donors are found. The number of digits read in this trial represent the number of people seen until three Type A donors are found. Repeat fifty times and average the fifty results to get an estimate of the average number of people who need to be seen.

Question 26

26. A clothing store tracks its inventory of red (R), green (G), and blue (B) clothing with the matrices below.



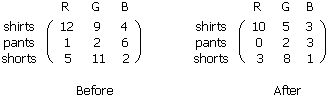
The matrix on the left shows the inventory before sales are recorded and the matrix on the right shows the inventory after sales have been recorded. Which of the following categories of clothing shows the greatest percent turnover?

There are two three by three matrices, one labeled before inventory and one labeled after inventory. Both matrices have rows labeled, from the top down, as shirts, pants, and shorts. The columns in both matrices are labeled, left to right, as red, green, and blue. The entries in the first row of the before inventory are twelve, nine, and four; in the second row one, two, and six; and in the third row five, eleven, and two. In the after matrix, the first row entries are ten, five, and three; the second row entries are zero, two, and three; and in the third row, three, eight, and one.

1. blue
2. shorts
3. red
4. pants

Answer to question 26

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0013) To find the percent turnover, find the number of pairs of pants in the inventory before sales were recorded: 1 + 2 + 6 = 9 and the number of pairs of pants in the inventory after sales were recorded: 0 + 2 + 3 = 5. The turnover is the difference, 4. To find the percent turnover, compare the turnover to the original inventory number: 4 ÷ 9 ≈ 44.4%. Comparing this figure to the results for blue (41.7%), shorts (33.3%), and red (27.8%), the percent turnover for pants was the greatest. Correct Response: D. (Objective 0013) To find the percent turnover, find the number of pairs of pants in the inventory before sales were recorded: one plus two plus six equals nine and the number of pairs of pants in the inventory after sales were recorded: zero plus two plus three equals five. The turnover is the difference, four. To find the percent turnover, compare the turnover to the original inventory number: four divided by nine approximately equals forty four point four percent. Comparing this figure to the results for blue (forty one point seven percent), shorts (thirty three point three percent), and red (twenty seven point eight percent), the percent turnover for pants was the greatest.

Question 27

27. Set X consists of one element selected from set R = {1, 2}, one element from set S = {3, 4, 5}, and one element from set T = {6, 7}. In how many ways can set X be chosen to include either the 4, the 6, or both?

1. 7
2. 8
3. 9
4. 10

Answer to question 27

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0013) Ways of choosing set X that meet the given criteria are {1, 3, 6}, {1, 4, 6}, {1, 4, 7}, {1, 5, 6}, {2, 3, 6}, {2, 4, 6}, {2, 4, 7}, and {2, 5, 6}. Alternatively, there are 2 × 3 × 2 = 12 possible sets, and only four of these do not contain a 4 or a 6: {1, 3, 7}, {1, 5, 7}, {2, 3, 7}, and {2, 5, 7}. Correct Response: B. (Objective 0013) Ways of choosing set X that meet the given criteria are left brace one comma three comma six right brace, left brace one comma four comma six right brace, left brace one comma four comma seven right brace, left brace one comma five comma six right brace, left brace two comma three comma six right brace, left brace two comma four comma six right brace, left brace two comma four comma seven right brace, and left brace two comma five comma six right brace. Alternatively, there are two times three times two equals twelve possible sets, and only four of these do not contain a four or a six: left brace one comma three comma seven right brace, left brace one comma five comma seven right brace, left brace two comma three comma seven right brace, and left brace two comma five comma seven right brace.

Question 28

28. Which of the following matrices is an identity matrix for matrix M below?

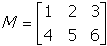
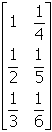
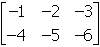
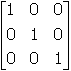
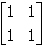
Answer to question 28

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0013) If the product of a given matrix M and matrix I is the original matrix M, I is an identity matrix for M. In this case, 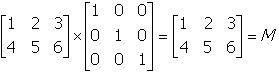. Therefore, is an identity matrix for matrix M. Note that 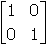is also an identity matrix for M because 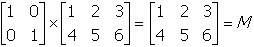. Correct Response: C. (Objective 0013) If the product of a given matrix M and matrix I is the original matrix M, I is an identity matrix for M. In this case, start two by three matrix first row first column one second column two third coulmn three. second row first column four second column five third column six end matrix times start three by three matrix first row first column one second column zero third column zero. second row first column zero second column one third column zero. third row first column zero second column zero third column one end matrix equals start two by three matrix first row first column one second column two third coulmn three. second row first column four second column five third column six end matrix equals upper M. Therefore, start three by three matrix first row first column one second column zero third column zero. second row first column zero second column one third column zero. third row first column zero second column zero third column one end matrix is an identity matrix for matrix M. Note that start two by two matrix first row first column one second column zero. second row first column zero second column one end matrix is also an identity matrix for M because start two by two matrix first row first column one second column zero. second row first column zero second column one end matrix times start two by three matrix first row first column one second column two third column three. second row first column four second column five third column six end matrix equals start two by three matrix first row first column one second column two third coulmn three. second row first column four second column five third column six end matrix equals upper M.

Question 29

29. Which of the following expressions represents the general relationship between the number of vertices, v, edges, e, and faces, f, in a planar graph, such as the one shown below?

1. e − v + f = 2
2. v − f + e = 2
3. e + v − f = 2
4. v − e + f = 2

Answer to question 29

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0013) In solving this problem, the examinee derives Euler's formula relating the number of vertices, edges, and faces of a planar graph to each other. In this case, there are 8 vertices (intersections), 12 edges (line segments), and 6 faces (including the outer unbounded face), numbers which are consistent with the equation v − e + f = 2. Correct Response: D. (Objective 0013) In solving this problem, the examinee derives Euler's formula relating the number of vertices, edges, and faces of a planar graph to each other. In this case, there are 8 vertices (intersections), 12 edges (line segments), and 6 faces (including the outer unbounded face), numbers which are consistent with the equation v minus e plus f equals two.

Question 30

30. Use the Venn diagram below to answer the question that follows.

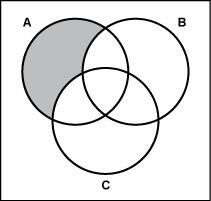
Which of the following expressions represents the shaded region in the Venn diagram shown?

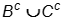
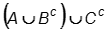
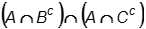
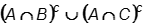
Answer to question 30

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0013) The expression 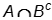represents the set of things in A but not in B. Similarly, 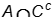represents the set of things in A but not in C. The intersection of these sets is the set of things in A which are neither in B nor in C. This statement describes the given diagram. Correct Response: C. (Objective 0013) The expression A intersection the complement of B represents the set of things in A but not in B. Similarly, A intersection complement of C represents the set of things in A but not in C. The intersection of these sets is the set of things in A which are neither in B nor in C. This statement describes the given diagram.

Question 31

31. Three faces of a rectangular prism have areas of 24, 32, and 48 square inches. If the lengths of the sides are all integers, what is the volume of the prism?

1. 96 in.³96 inches cubed
2. 192 in.³192 inches cubed
3. 288 in.³288 inches cubed
4. 384 in.³384 inches cubed

Answer to question 31

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0014) Knowledge of number theory (factors) and geometry (volume) can be used to solve this problem. Possible side lengths of the 24-square-inch face, the 32-square-inch face, and the 48-square-inch face are 1, 2, 3, 4, 6, 8, 12, and 24 inches; 1, 2, 4, 8, 16, and 32 inches; and 1, 2, 3, 4, 6, 8, 12, 16, 24, and 48 inches, respectively. Start with the 32-square-inch face, since that has the fewest factors: 32 cannot work, since neither of the other two faces can have a side 32 inches long. If the dimensions of the 32-square-inch face are 16 × 2, then the dimensions of the prism must be 16 × 2, 16 × 3, and 2 × 3, but there is not a 6-square-inch face. If the dimensions of the 32-square-inch face are 8 × 4, possible dimensions of the prism are 8 × 4, 8 × 3, and 3 × 4 or 8 × 4, 8 × 6, and 4 × 6. Only the latter dimensions are consistent with face areas of 24, 32, and 48 square inches. Thus, the volume of the prism is 8 × 6 × 4 = 192 cubic inches. Correct Response: B. (Objective 0014) Knowledge of number theory (factors) and geometry (volume) can be used to solve this problem. Possible side lengths of the 24-square-inch face, the 32-square-inch face, and the 48-square-inch face are 1, 2, 3, 4, 6, 8, 12, and 24 inches; 1, 2, 4, 8, 16, and 32 inches; and 1, 2, 3, 4, 6, 8, 12, 16, 24, and 48 inches, respectively. Start with the 32-square-inch face, since that has the fewest factors: 32 cannot work, since neither of the other two faces can have a side 32 inches long. If the dimensions of the 32-square-inch face are 16 times 2, then the dimensions of the prism must be 16 times 2, 16 times 3, and 2 times 3, but there is not a 6-square-inch face. If the dimensions of the 32-square-inch face are 8 times 4, possible dimensions of the prism are 8 times 4, 8 times 3, and 3 times 4 or 8 times 4, 8 times 6, and 4 times 6. Only the latter dimensions are consistent with face areas of 24, 32, and 48 square inches. Thus, the volume of the prism is 8 times 6 times 4 equals 192 cubic inches.

Question 32

32. Companies that sell life insurance set their premium rates using actuarial tables that provide data on the life expectancies of people of different ages. This process of rate setting is based on which of the following branches of mathematics?

1. probability
2. linear algebra
3. calculus
4. number theory

Answer to question 32

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0014) An actuarial table or life table is a table that provides, for any given age, the probability that a person of that age will die before their next birthday. It enables life insurance companies to calculate policy rates based on the life expectancies of the people buying the insurance. Correct Response: A. (Objective 0014) An actuarial table or life table is a table that provides, for any given age, the probability that a person of that age will die before their next birthday. It enables life insurance companies to calculate policy rates based on the life expectancies of the people buying the insurance.

Question 33

33. Which of the following types of representations provides the most effective way to show the common factors of three different numbers?

1. number line
2. circle graph
3. Venn diagram
4. function map

Answer to question 33

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0014) The Venn diagram below shows all the factors of 18, 24, and 36, including their common factors. The factors 1, 2, 3, and 6, which are common to all three numbers, are shown in the intersection of the three circles. The factors 9 and 18 that are common only to 18 and 36 show where those two circles intersect; and the factors 4 and 12 that are common to only 24 and 36 show where those two circles intersect. The factors 1, 2, 3, and 6, which are common to all three numbers, are shown in the intersection of the three circles. The factors 9 and 18 that are common only to 18 and 36 show where those two circles intersect; and the factors 4 and 12 that are common to only 24 and 36 show where those two circles intersect. Correct Response: C. (Objective 0014) The Venn diagram below shows all the factors of 18, 24, and 36, including their common factors. The factors 1, 2, 3, and 6, which are common to all three numbers, are shown in the intersection of the three circles. The factors 9 and 18 that are common only to 18 and 36 show where those two circles intersect; and the factors 4 and 12 that are common to only 24 and 36 show where those two circles intersect. venn diagram showing the common factors of eighteen twenty four and thirty six. Three overlapping circles with number inside specific parts to show the relationship. The factors one, two, three, and six, which are common to all three numbers, are shown in the intersection of the three circles. The factors nine and eighteen that are common only to eighteen and thirty six show where those two circles intersect; and the factors four and twelve that are common to only twenty four and thirty six show where those two circles intersect

Question 34

34. Which of the following equations can be used to solve the problem below?

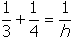
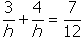
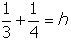
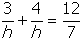
Answer to question 34

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0014) The number of jobs completed, j, can be calculated as the product of the number of hours, h, worked, and the rate, r, of work in jobs per hour, so j = h × r. In this case, the total number of jobs completed by the workers together (j = 1) equals the sum of the work done by each worker, or 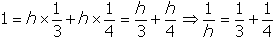, which can then be solved for h. Alternatively, reason directly that 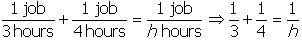. Correct Response: A. (Objective 0014) The number of jobs completed, j, can be calculated as the product of the number of hours, h, worked, and the rate, r, of work in jobs per hour, so j equals h multiplied by r. In this case, the total number of jobs completed by the workers together open parenthesis j equals 1 close parenthesis equals the sum of the work done by each worker, or one equals h times one third plus h times one fourth equals h over three baseline plus h over four baseline leads to one over h baseline equals one third plus one fourth, which can then be solved for h. Alternatively, reason directly that one job over three hours baseline plus one job over four hours baseline equals one job over h hours baseline leads to one third plus one fourth equals one over h.

Question 35

35. Computers use logical operators based on the binary number system and combinations of AND and OR circuits to perform the steps written into their software. Their language of logic is most directly based on:

1. Boolean algebra.
2. the Binomial Theorem.
3. modular arithmetic.
4. Euler circuits.

Answer to question 35

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0014) George Boole (1815–1864) believed that mathematics is a system of logical thought that could be used to show relationships between classes of things. He devised a method of expressing statements symbolically and manipulating them algebraically. Boolean logic can be applied to any statement that has exactly two outcomes: true/false, belonging/not belonging, etc. The binary number system is used to express these outcomes through electrical flow (1 = true, belongs, etc.) or no flow (0 = false, does not belong, etc.) through the computer's circuits. Correct Response: A. (Objective 0014) George Boole (eighteen fifteen to eighteen sixty four) believed that mathematics is a system of logical thought that could be used to show relationships between classes of things. He devised a method of expressing statements symbolically and manipulating them algebraically. Boolean logic can be applied to any statement that has exactly two outcomes: true or false, belonging or not belonging, etcetera. The binary number system is used to express these outcomes through electrical flow (one equals true, belongs, etcetera) or no flow (zero equals false, does not belong, etcetera) through the computer's circuits.

Question 36

36. Which of the following strategies would be most effective in promoting students' understanding and retention of new vocabulary associated with a specific unit of study?

1. using weekly quizzes to assess students' knowledge of newly introduced vocabulary
2. having students look up definitions of new vocabulary in both a textbook glossary and a standard dictionary
3. providing multiple opportunities for students to use new vocabulary in discussions and in their work
4. encouraging students to enter new vocabulary in a personal dictionary of words they find interesting or useful

Answer to question 36

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: C. (Objective 0015) Vocabulary knowledge is both a key indicator and predictor of comprehension ability. Vocabulary knowledge and concept development are closely interrelated; discipline-specific vocabulary development involves concept learning, and concept learning supports academic vocabulary development. Vocabulary learning is an incremental process; more exposures to and opportunities to use new vocabulary in context result in greater depth of understanding. Providing students with multiple opportunities to use the vocabulary from a new unit of study in discussions and in their writing promotes the students' vocabulary learning while simultaneously strengthening their understanding of associated concepts. Correct Response: C. (Objective 0015) Vocabulary knowledge is both a key indicator and predictor of comprehension ability. Vocabulary knowledge and concept development are closely interrelated; discipline-specific vocabulary development involves concept learning, and concept learning supports academic vocabulary development. Vocabulary learning is an incremental process; more exposures to and opportunities to use new vocabulary in context result in greater depth of understanding. Providing students with multiple opportunities to use the vocabulary from a new unit of study in discussions and in their writing promotes the students' vocabulary learning while simultaneously strengthening their understanding of associated concepts.

Question 37

37. A student is reading a mathematics text and encounters a section that does not make sense to the student. Which of the following strategies would be most appropriate for the student to use in this situation to improve and repair reading comprehension?

1. applying contextual analysis to deduce the meaning of any unfamiliar words encountered in the section
2. increasing reading rate and fluency by skipping over the parts of the section that are most confusing
3. identifying the topic sentences throughout the section and analyzing each of the sentences syntactically
4. making connections between concepts previously read or learned and those presented in the section

Answer to question 37

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0015) As described, the student's comprehension difficulty is limited to a particular section of the text and therefore the student has understood the sections of text that preceded the problematic section. Typically, the concepts and information presented in different sections of a mathematics text are related and are organized so that foundational concepts and information are presented first. Making connections between concepts in the confusing section and concepts previously learned in earlier sections is an effective metacognitive strategy for actively constructing meaning during reading. The concepts previously presented are likely to provide relevant background information to support comprehension of the difficult section. Correct Response: D. (Objective 0015) As described, the student's comprehension difficulty is limited to a particular section of the text and therefore the student has understood the sections of text that preceded the problematic section. Typically, the concepts and information presented in different sections of a mathematics text are related and are organized so that foundational concepts and information are presented first. Making connections between concepts in the confusing section and concepts previously learned in earlier sections is an effective metacognitive strategy for actively constructing meaning during reading. The concepts previously presented are likely to provide relevant background information to support comprehension of the difficult section.

Question 38

38. A mathematics teacher regularly conducts a "graphic walk" through a text prior to assigning the text for reading. During the "walk," the teacher leads students in analyzing each graphic in the text and noting in the text margins or on a sticky note what information the graphic conveys. Later, when students read the text, the teacher has them look for statements or claims by the author that are supported by the information in the graphic. The teacher's practice of conducting "graphic walks" supports students' content-area reading development primarily by promoting their ability to:

1. use graphic organizers to analyze a text's overall structure.
2. summarize key information in a text without plagiarizing an author's words.
3. make inferences about ideas or concepts described in a text.
4. integrate and evaluate information presented in different formats in a text.

Answer to question 38

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: D. (Objective 0015) Graphics and text can present similar information while emphasizing different details because of their varying formats. Helping students make explicit connections between the information presented in the graphics and written discourse in a text promotes their critical thinking and encourages them to integrate the information from diverse formats as they read rather than treating graphics as extraneous information that can be ignored. Correct Response: D. (Objective 0015) Graphics and text can present similar information while emphasizing different details because of their varying formats. Helping students make explicit connections between the information presented in the graphics and written discourse in a text promotes their critical thinking and encourages them to integrate the information from diverse formats as they read rather than treating graphics as extraneous information that can be ignored.

Question 39

39. Several students in a mathematics class demonstrate strong comprehension of the subject matter and related terminology during class discussions. When called on to read aloud a section of a text in class, they can read accurately and at an appropriate rate. However, these same students demonstrate little comprehension of what they have read, even when they read a text silently. The mathematics teacher could best address the content-area reading needs of these students by modeling and providing the students with practice in which of the following components of reading as part of content-area lessons?

1. applying morphological knowledge
2. self-monitoring/questioning
3. activating prior knowledge
4. print processing/decoding

Answer to question 39

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: B. (Objective 0015) The students in this scenario demonstrate proficiency in many key components and processes involved in reading—they have adequate decoding and reading fluency skills, content-specific vocabulary knowledge, and background knowledge of the subject matter. Yet, when they read a text orally or silently, they do not comprehend what they are reading. Self-monitoring/questioning is a metacognitive strategy in which readers actively monitor whether or not they understand what they are reading. If they encounter a problem, they immediately seek to repair comprehension by self-questioning and rereading as necessary. Correct Response: B. (Objective 0015) The students in this scenario demonstrate proficiency in many key components and processes involved in reading—they have adequate decoding and reading fluency skills, content-specific vocabulary knowledge, and background knowledge of the subject matter. Yet, when they read a text orally or silently, they do not comprehend what they are reading. Self-monitoring/questioning is a metacognitive strategy in which readers actively monitor whether or not they understand what they are reading. If they encounter a problem, they immediately seek to repair comprehension by self-questioning and rereading as necessary.

Question 40

40. A mathematics teacher would like to have students read a complex informational text that is closely related to the current unit of study. However, the teacher is aware that the text is written above the reading proficiency level of many students in the class. Which of the following approaches to using the text would be most effective in both making the text's content accessible to all students and promoting the students' content-area reading development?

1. helping the students deconstruct the text's complex academic language and concepts during a whole-class close reading of the text
2. preparing a detailed written outline of the text's key ideas and details for the students to read and study rather than assigning the original text
3. selecting a different text that addresses more basic concepts and is written at a lower reading proficiency level so that the students can read it independently
4. providing a detailed oral preview of the text's key concepts, having the students read the text silently, then providing an oral review of the concepts as reinforcement

Answer to question 40

Answer Enter to expand or collapse answer. Answer expanded

Correct Response: A. (Objective 0015) To promote students' college and career readiness, content-area educators must prepare students to construct meaning from complex discipline-specific texts. Reading research has shown that if students are "spoon-fed" the content of complex texts, they never develop the skills and strategies necessary to grapple with complex texts on their own. Instead of "spoon feeding," content-area teachers need to use a gradual release of responsibility approach. Close reading involves reading and rereading a complex text, section by section, during which the teacher models and provides students with opportunities to practice key content-area reading skills, such as how to deconstruct syntactically complex sentences, use various word-learning strategies, and apply relevant comprehension strategies to unlock the meaning and evaluate the significance of the text's content. Correct Response: A. (Objective 0015) To promote students' college and career readiness, content-area educators must prepare students to construct meaning from complex discipline-specific texts. Reading research has shown that if students are "spoon-fed" the content of complex texts, they never develop the skills and strategies necessary to grapple with complex texts on their own. Instead of "spoon feeding," content-area teachers need to use a gradual release of responsibility approach. Close reading involves reading and rereading a complex text, section by section, during which the teacher models and provides students with opportunities to practice key content-area reading skills, such as how to deconstruct syntactically complex sentences, use various word-learning strategies, and apply relevant comprehension strategies to unlock the meaning and evaluate the significance of the text's content.